Mensuration : Length, Area, and Volume : Area of Parts of Circle : Sector and Segment

maths > mensuration-high

Area of Parts of Circle : Sector and Segment

what you'll learn...

Overview

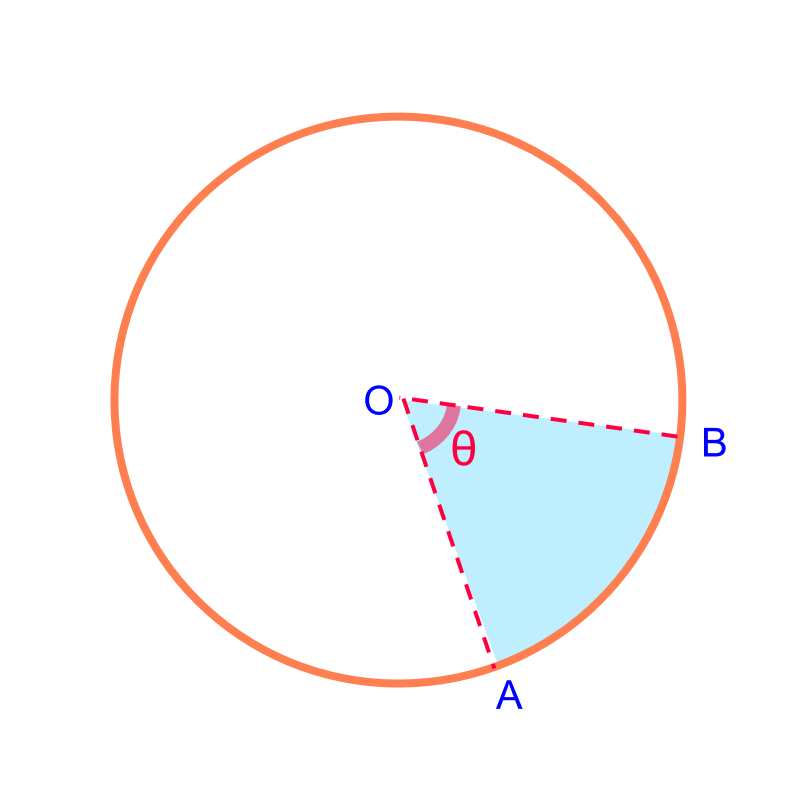sector of a circle Arc Length and Area of a Sector: The arc-length and area are proportion to the angle subtended by the sector.

Arc length of a sector of $θ^{\circ}$ $theta^@$ angle $= 2 π r \times \frac{θ}{360}$ $=2 pi r xx theta/360$

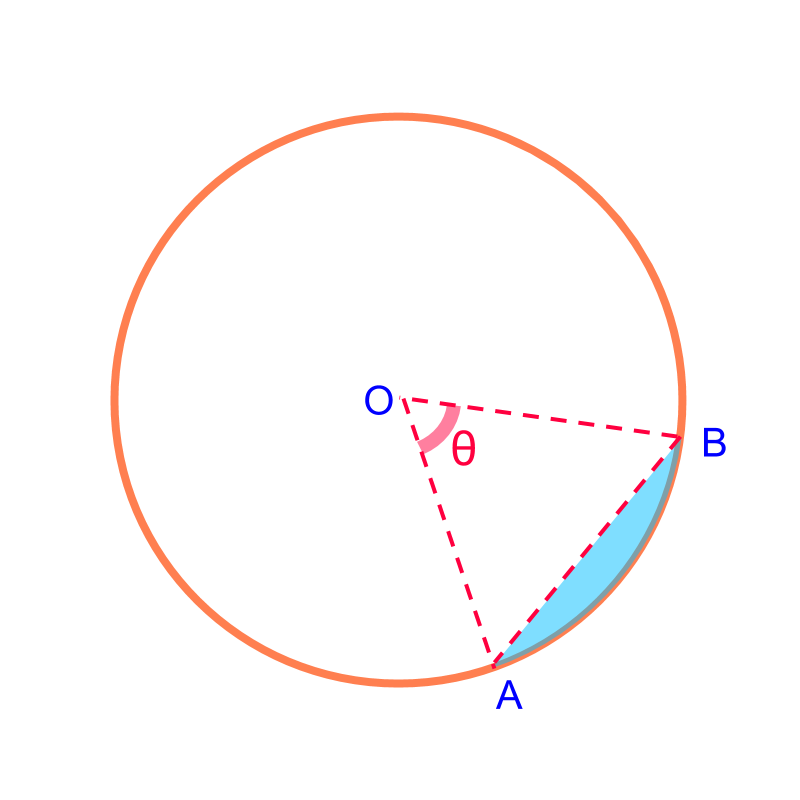Area of a sector of $θ^{\circ}$ $theta^@$ angle $= π r^{2} \times \frac{θ}{360}$ $=pi r^2 xx theta/360$ segment of a circle Area of a Segment: is the difference between area of sector and the triangle.
Area of a Segment of $θ^{\circ}$ $theta^@$ angle = area of the corresponding sector $-$ $-$ the triangle

recap circle

The perimeter and area of a circle of radius $r$ $r$ is " $2 π r$ $2pi r$ and $π r^{2}$ $pi r^2$ "

The angle subtended by a circle on the center is " $360^{\circ}$ $360^@$ "

sector

Consider the sector of a circle shown in the figure. The parameters required to define the sector are "the radius and the angle subtended by the sector"

We learned that

Perimeter of a circle is $2 π r$ $2 pi r$

Area of a circle is $π r^{2}$ $pi r^2$

Angle subtended by the entire circle is $360^{\circ}$ $360^@$

By the symmetry in the circle, it is found that the sector of angle $θ$ $theta$ has

Arc length of a sector of $θ$ $theta$ is $2 π r \times \frac{θ}{360}$ $2 pi r xx theta/360$ . That is, the ratio of angle of the sector to full-angle is the ratio of perimeter of the sector to that of the full-circle.

Area of a sector of $θ$ $theta$ is $π r^{2} \frac{θ}{360}$ $pi r^2 theta/360$ . That is, the ratio of angle of the sector to full-angle is the ratio of area of the sector to that of the full-circle.

measures of sector

Consider the sector given in figure. The arc length of minor arc $A B$ $AB$ is " $2 π r \times \frac{θ}{360}$ $2 pi r xx theta/360$ "

The perimeter of the shaded sector $O A B$ $OAB$ is curve plus two radius length = $2 π r \times \frac{θ}{360} + 2 r$ $2 pi r xx theta/360 + 2r$

The area of the shaded sector $O A B$ $OAB$ is " $π r^{2} \times \frac{θ}{360}$ $pi r^2 xx theta/360$ ".

The area of the shaded segment is "Area of triangle $O A B$ $OAB$ is subtracted from area of sector $O A B$ $OAB$ "

What is the area of a sector of $180^{\circ}$ $180^@$ and segment of $180^{\circ}$ $180^@$ in a circle?

The answer is "area of sector equals the area of segment $= π r$ $= pi r$ "

summary

Arc Length and Area of a Sector: sector of a circle The arc-length and area are proportion to the angle subtended by the sector.

Arc length of a sector of $θ^{\circ}$ $theta^@$ angle $= 2 π r \times \frac{θ}{360}$ $=2 pi r xx theta/360$

Area of a sector of $θ^{\circ}$ $theta^@$ angle $= π r^{2} \times \frac{θ}{360}$ $=pi r^2 xx theta/360$

Area of a Segment: is the difference between area of sector and the triangle. segment of a circle Area of a Segment of $θ^{\circ}$ $theta^@$ angle = area of the corresponding sector $-$ $-$ the triangle

Outline

The outline of material to learn Mensuration : Length, Area, and Volume is as follows.

Note 1: click here for the detailed overview of Mensuration High

Note 2: click here for basics of mensuration, which is essential to understand this.

• Basics of measurement

→ Summary of Measurement Basics

→ Measurement by superimposition

→ Measurement by calculation

→ Measurement by equivalence

→ Measurement by infinitesimal pieces

→ Cavalieri's Principle (2D)

→ Cavalieri's Principle (3D)

• Perimeter & Area of 2D shapes

→ Circumference of Circles

→ Area of Circles

• Surface area & Volume of 3D shapes

→ Prisms : Surface Area & Volume

→ Pyramids : Surface Area & Volume

→ Cone : Surface Area & Volume

→ Sphere : Surface Area & Volume

• Part Shapes

→ Understanding part Shapes

→ Circle : Sector and Segment

→ Frustum of a Cone