Mensuration : Length, Area, and Volume : Pyramids : Surface Area and Volume

maths > mensuration-high

Pyramids : Surface Area and Volume

what you'll learn...

Overview

Surface Area and Volume of pyramid: surface area of a pyramid Total surface area of the pyramid
$=$ $=$ area of the base-surface $+$ $+$ sum of the area of the side-faces volume of a pyramid Volume of the pyramid
$= \frac{1}{3} \times area of the base-face \times height$ $= 1/3 xx text( area of the base-face ) xx text( height)$

pyramid

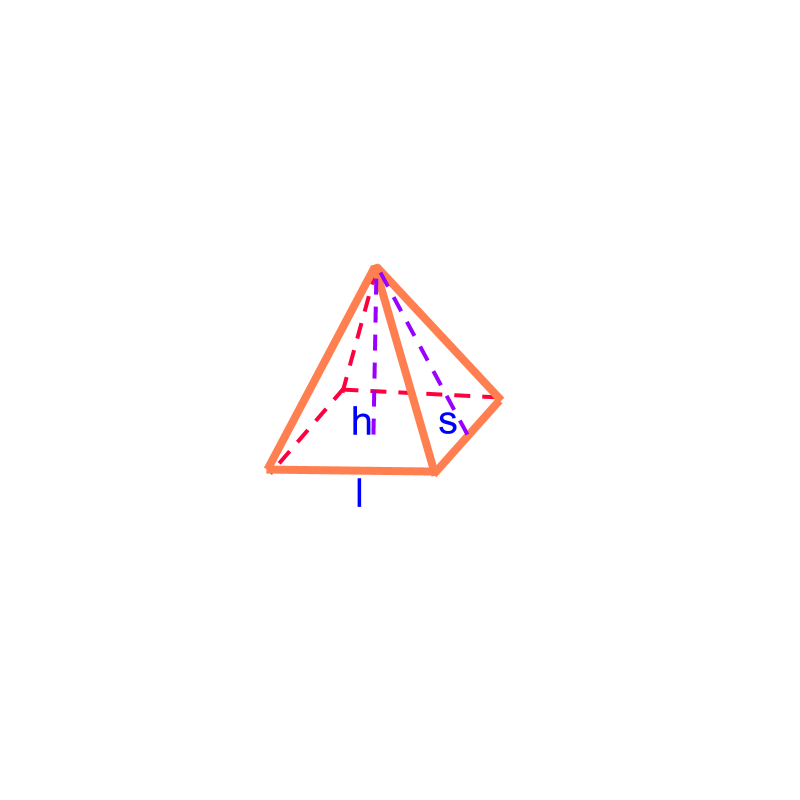
The shape shown in the figure is "a pyramid". Pyramid is a 3D solid shape with a 2D-polygon base at the bottom with triangular faces on the sides converging to a single point on the top . The base can be any 2D-polygon, for example, square, rectangle, triangle, etc, and the pyramids are square-pyramid, rectangular-pyramid, triangular-pyramid respectively.

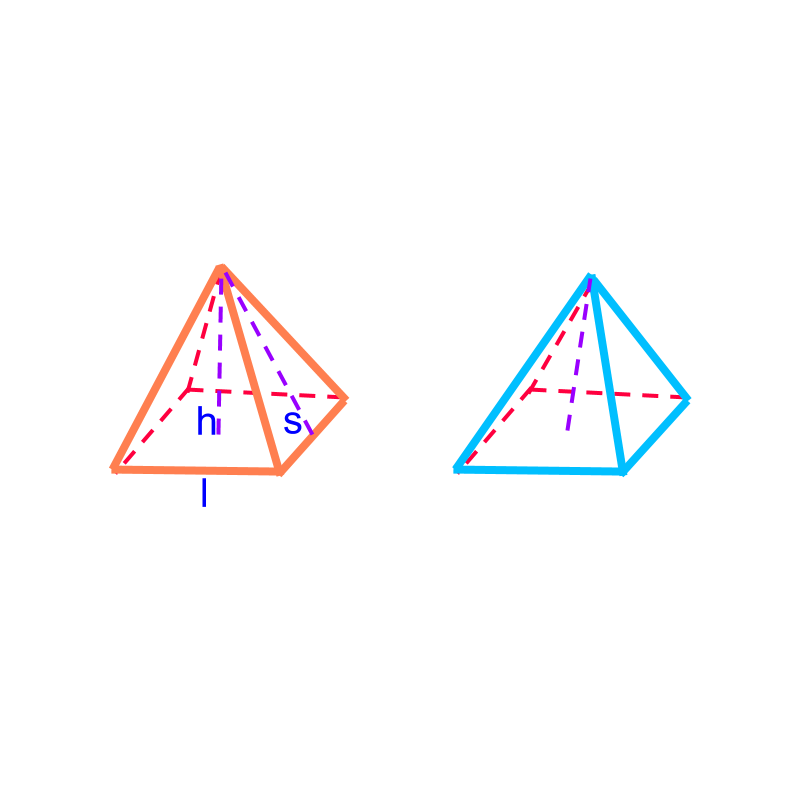
We consider only right-pyramids with its axis at right angle to the base-surface. The oblique-pyramids have the angle between its axis and the base is not a right-angle. A right pyramid is shown in orange. And an oblique pyramid is shown in blue.

surface area

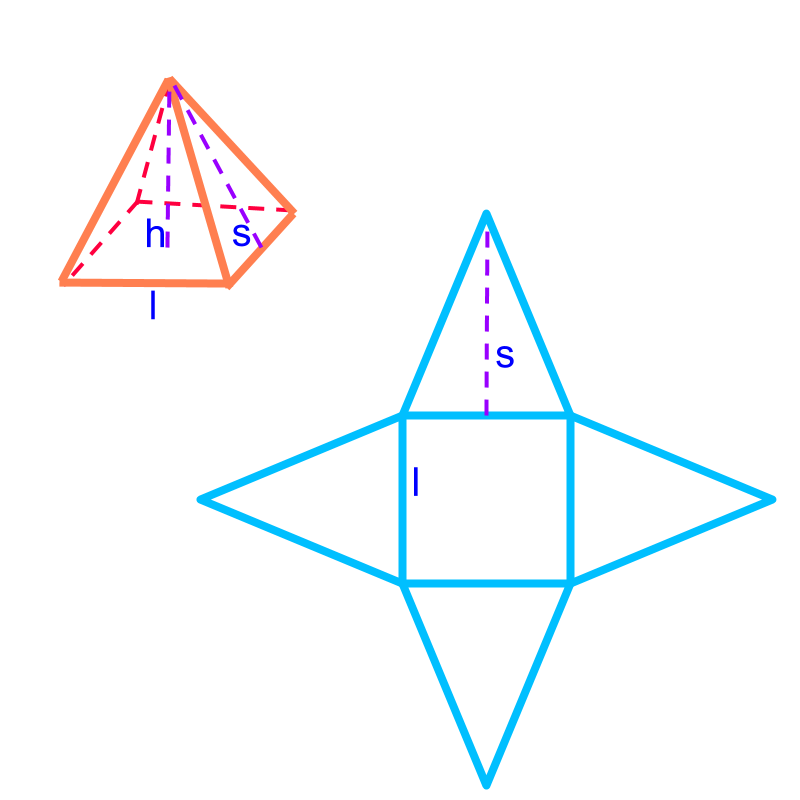
The surface area of the square-pyramid of side $a$ $a$ and height $h$ $h$ is

$area of square$ $text( area of square )$ $+ 4 \times area of triangles$ $+ 4 xx text( area of triangles)$ or

$area of square$ $text( area of square )$ $+ \frac{1}{2} perimeter of base-surface \times (s l a n t h e i g h t)$ $+ 1/2 text( perimeter of base-surface) xx ( slant height)$

Note: A generic pyramid consists of

base-surface a 2D-polygon, (in this problem a square)

a set of triangular faces, (in this problem $5$ $5$ triangles of base $a$ $a$ and height $s$ $s$ ).

$s$ $s$ is the slant height computed as $\sqrt{a^{2} / 4 + h^{2}}$ $sqrt(a^2//4 + h^2)$ .

A pyramid of height $h$ $h$ is shown in orange. The triangular faces are shown in blue. Total surface area of the pyramid

$=$ $=$ area of the base-surface $+$ $+$ sum of the area of the triangular faces

volume of square pyramid

The volume of the square-pyramid of side $l$ $l$ and height $h$ $h$ is $\frac{1}{3} \times area of base-surface$ $1/3 xx text( area of base-surface )$ $\times height$ $xx text( height)$

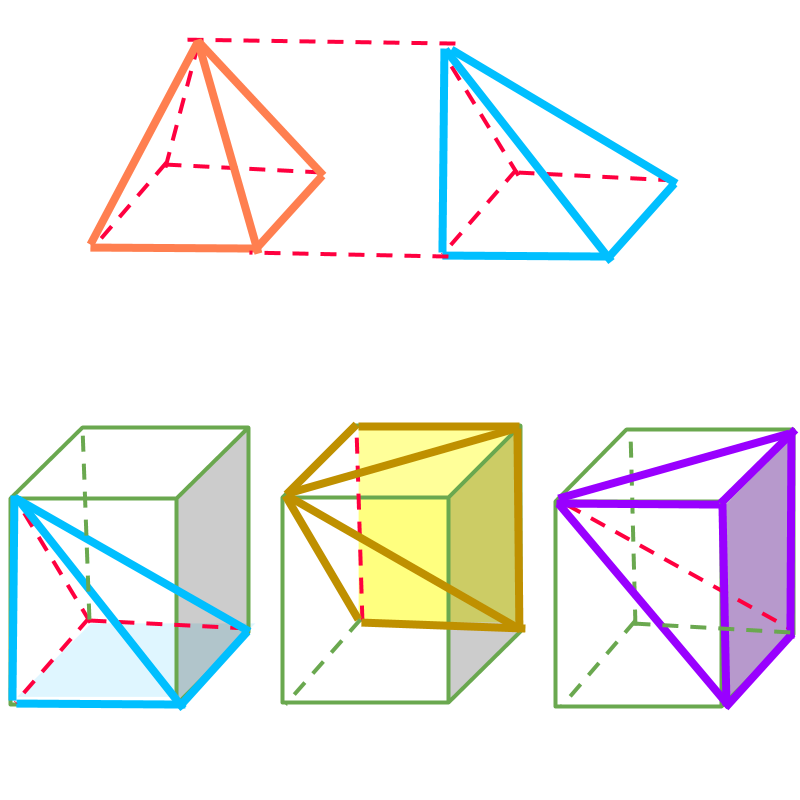
Note: As per the Cavalieri's principle in 3D, the pyramid is equivalently represented by the oblique-pyramid in blue.

The modified-pyramid fits into a cuboid. Each of the pyramids in blue, green, and purple, equal in volume, fit into the cuboid, and spans the entire cuboid. So, each fills exactly one-third of the cuboid as shown.

A square-pyramid of height $h$ $h$ is shown in orange. As per the Cavalieri's principle in 3D, the pyramid is equivalently represented by the oblique-pyramid in blue.

The modified-pyramid fits into a cuboid. Each of the pyramids, shown on the lower half of the figure, in blue, green, and purple, are

• equal in volume,

• fit into the cuboid, and

• spans the entire cuboid. So, each fills exactly one-third of the cuboid as shown.

Volume of the pyramid

$=$ $=$ volume of the modified pyramid with identical cross sectional area along vertical axis

$= \frac{1}{3} \times area of the base \times height$ $= 1/3 xx text( area of the base ) xx text( height)$

volume of any pyramid

The volume of the pentagonal-pyramid shown in the figure is $\frac{1}{3} \times area of base-surface$ $1/3 xx text( area of base-surface )$ $\times height$ $xx text( height)$

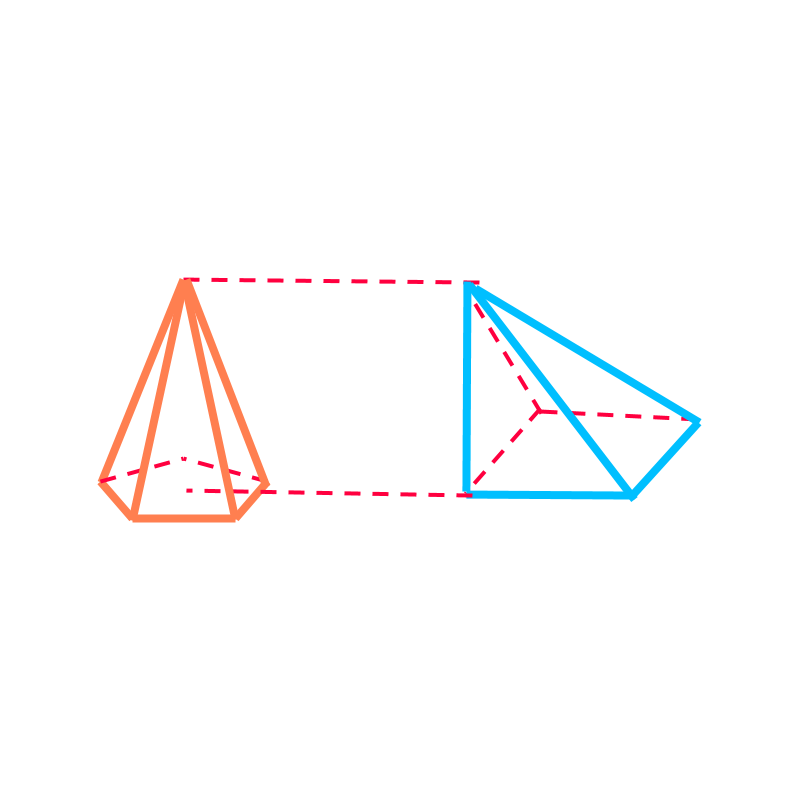
A pentagonal-pyramid is shown in orange. As per the Cavalieri's principle in 3D, the pyramid is equivalently represented by the oblique-pyramid in blue.

The modified-pyramid has

• the area at the bottom surface equal to the pentagonal pyramid

• the height equal to the pentagonal pyramid.

• the cross-sectional area at any point along vertical axis equals to the same of pentagonal pyramid

Volume of the pyramid

$=$ $=$ volume of the modified pyramid with identical cross sectional area along vertical axis

$= \frac{1}{3} \times area of the base \times height$ $= 1/3 xx text( area of the base ) xx text( height)$

What is the volume of a pyramid with base area $30 c m^{2}$ $30cm^2$ and height $2$ $2$ cm? Volume can be computed without specifying what type of pyramid is that. Volume $= \frac{1}{3}$ $=1/3$ base-area $\times$ $xx$ height $= 20 c m^{3}$ $=20cm^3$

summary

Outline

The outline of material to learn Mensuration : Length, Area, and Volume is as follows.

Note 1: click here for the detailed overview of Mensuration High

Note 2: click here for basics of mensuration, which is essential to understand this.

• Basics of measurement

→ Summary of Measurement Basics

→ Measurement by superimposition

→ Measurement by calculation

→ Measurement by equivalence

→ Measurement by infinitesimal pieces

→ Cavalieri's Principle (2D)

→ Cavalieri's Principle (3D)

• Perimeter & Area of 2D shapes

→ Circumference of Circles

→ Area of Circles

• Surface area & Volume of 3D shapes

→ Prisms : Surface Area & Volume

→ Pyramids : Surface Area & Volume

→ Cone : Surface Area & Volume

→ Sphere : Surface Area & Volume

• Part Shapes

→ Understanding part Shapes

→ Circle : Sector and Segment

→ Frustum of a Cone