Trigonometry (Introduction) : Trigonometric Ratios for Complementary Angles

maths > trigonometry

Trigonometric Ratios for Complementary Angles

what you'll learn...

Overview

» Quickly follow that the adjacent and opposite sides swap for complementary angles... no need to memorize.

      $\sin (90 - θ) = \cos θ$ $sin(90-theta) = cos theta$
      $\cos (90 - θ) = \sin θ$ $cos(90-theta) = sin theta$
      $\tan (90 - θ) = \cot θ$ $tan(90-theta) = cot theta$

      $\csc (90 - θ) = \sec θ$ $csc(90-theta) = sec theta$
      $\sec (90 - θ) = \csc θ$ $sec(90-theta) = csc theta$
      $\cot (90 - θ) = \tan θ$ $cot(90-theta) = tan theta$

Proof

The complementary angle of $θ$ $theta$ is $90 - θ$ $90-theta$ or the angle that completes the given angle to a right angle.

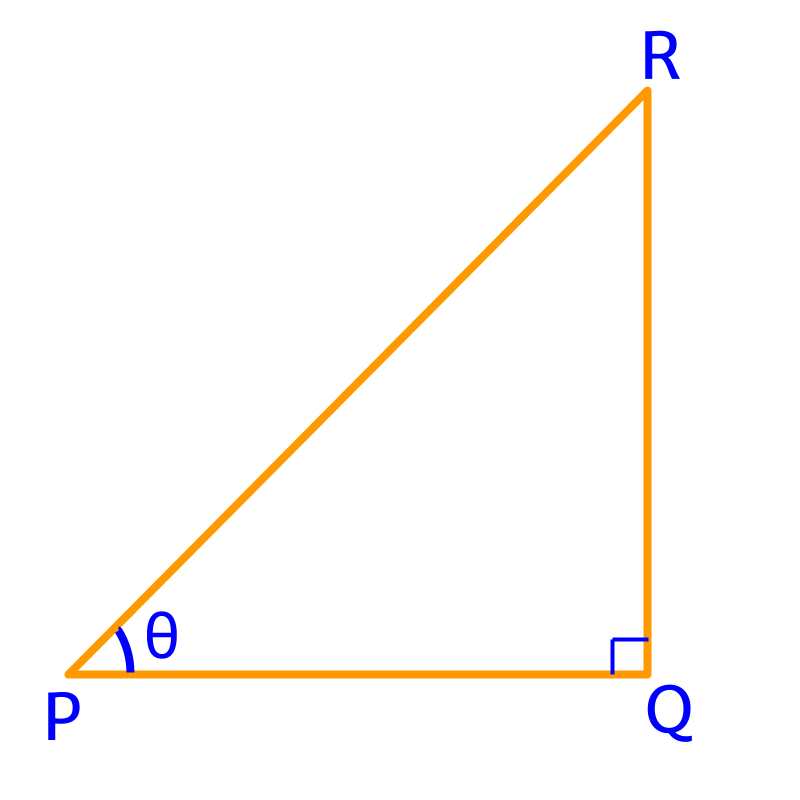
In the right angled triangle shown in figure, if $∠ P = θ$ $/_P = theta$ then

$∠ R = 90 - θ$ $/_R = 90-theta$ which is the complementary angle of $θ$ $theta$

In the right triangle shown in figure, the opposite side of $∠ P$ $/_P$ is $Q R$ $QR$ .

In the right triangle shown in figure, the adjacent side of $∠ R$ $/_R$ is $Q R$ $QR$ .

A right angled triangle is shown in figure.

Opposite of $∠ θ$ $/_theta$ = Adjacent of $∠ (90 - θ)$ $/_(90-theta)$
Adjacent of $∠ θ$ $/_theta$ = Opposite of $∠ (90 - θ)$ $/_(90-theta)$

The trigonometric ratios of $90 - θ$ $90-theta$ can be expressed in terms of trigonometric ratios of $θ$ $theta$ or vice versa.

From the figure
$\sin θ = \frac{R Q}{P R}$ $sin theta = (RQ)/(PR)$
$\cos θ = \frac{P Q}{P R}$ $cos theta = (PQ)/(PR)$
$\sin (90 - θ) = \frac{P Q}{P R}$ $sin (90-theta) = (PQ)/(PR)$
$\cos (90 - θ) = \frac{R Q}{P R}$ $cos (90-theta) = (RQ)/(PR)$

Comparing the above, it is concluded that
$\sin (90 - θ) = \cos θ$ $sin (90-theta) = cos theta$
$\cos (90 - θ) = \sin θ$ $cos (90-theta) = sin theta$

tan and cot

It is noted that the two angles in a right angles triangle are complementary angles and the trigonometric ratios of one angle are related to the trigonometric ratios of the other angle.

That is

• hypotenuse is common for both the angles

• opposite side for an angle is the adjacent side for the other angle

• adjacent side for an angle is the opposite side for the other angle

This relation is used to derived Trigonometric Ratios for Complementary Angles .

Trigonometric Ratios for Complementary Angles:
$\sin (90 - θ) = \cos θ$ $sin(90-theta) = cos theta$
$\cos (90 - θ) = \sin θ$ $cos(90-theta) = sin theta$

The trigonometric ratios of $90 - θ$ $90-theta$ can be expressed in terms of trigonometric ratios of $θ$ $theta$ or vice versa.

From the figure
$\tan θ = \frac{R Q}{P Q}$ $tan theta = (RQ)/(PQ)$
$\cot θ = \frac{P Q}{R Q}$ $cot theta = (PQ)/(RQ)$
$\tan (90 - θ) = \frac{P Q}{R Q}$ $tan (90-theta) = (PQ)/(RQ)$
$\cot (90 - θ) = \frac{R Q}{P Q}$ $cot (90-theta) = (RQ)/(PQ)$

Comparing the above, it is concluded that
$\tan (90 - θ) = \cot θ$ $tan (90-theta) = cot theta$
$\cot (90 - θ) = \tan θ$ $cot (90-theta) = tan theta$

secant and cosecant

The trigonometric ratios of $90 - θ$ $90-theta$ can be expressed in terms of trigonometric ratios of $θ$ $theta$ or vice versa.

From the figure
$\csc θ = \frac{P R}{R Q}$ $csc theta = (PR)/(RQ)$
$\sec θ = \frac{P R}{P Q}$ $sec theta = (PR)/(PQ)$
$\csc (90 - θ) = \frac{P R}{P Q}$ $csc (90-theta) = (PR)/(PQ)$
$\sec (90 - θ) = \frac{P R}{R Q}$ $sec (90-theta) = (PR)/(RQ)$

Comparing the above, it is concluded that
$\csc (90 - θ) = \sec θ$ $csc(90-theta) = sec theta$
$\sec (90 - θ) = \csc θ$ $sec(90-theta) = csc theta$

examples

Does $\sin 36$ $sin 36$ equal $\sin 54$ $sin 54$ or $\cos 54$ $cos 54$ ?
The answer is ' $\cos 54$ $cos 54$ ', as $\sin 36$ $sin 36$ $= \sin (90 - 54)$ $= sin(90-54)$ $= \cos 54$ $= cos 54$

$\frac{\tan 75}{\cot 15} = ?$ $(tan 75) / (cot 15) = ?$
The answer is ' $1$ $1$ ' As $\cot 15$ $cot 15$ $= \tan (90 - 15)$ $=tan(90-15)$ $= \tan 75$ $=tan 75$ .

Simplify $\sin 36 - \cos 36 + \sin 54$ $sin 36 - cos 36 + sin 54$ .
The answer is ' $\sin 36$ $sin 36$ '.

Summary

Quickly follow that the adjacent and opposite sides swap for complementary angles... no need to memorize.

$\sin (90 - θ) = \cos θ$ $sin(90-theta) = cos theta$

$\cos (90 - θ) = \sin θ$ $cos(90-theta) = sin theta$

$\tan (90 - θ) = \cot θ$ $tan(90-theta) = cot theta$

$\csc (90 - θ) = \sec θ$ $csc(90-theta) = sec theta$

$\sec (90 - θ) = \csc θ$ $sec(90-theta) = csc theta$

$\cot (90 - θ) = \tan θ$ $cot(90-theta) = tan theta$

Outline

The outline of material to learn "Basics of Trigonometry" is as follows.

• Detailed outline of "trigonometry".

→ Basics - Angles

→ Basics - Triangles

→ Importance of Right Angled Triangle

→ Trigonometric Ratio (Basics)

→ Triangular Form of Trigonometric Ratios

→ Introduction to Standard Angles

→ Trigonometric Ratio of Standard Angles

→ Trigonometric Identities

→ Trigonometric Ratios of Complementary Angles