Fractions : Fractions: Dividing a Group

maths > fractions

Fractions: Dividing a Group

what you'll learn...

overview

This page explains that fraction can be a part of a whole or can be a part of a group. In the latter case, a fraction represents the number of objects / items out of a total number of objects.
In this, fraction $\frac{p}{q}$ $p/q$ represents

$p$ $p$ number of items, for every $q$ $q$ number of items in the whole group.

one or many

There are $5$ $5$ flowers in the picture.

$fraction part of group$

Consider the enclosed picture. The total number of cars is 6. The number of red cars is 1. This is represented as a fraction $\frac{1}{6}$ $1/6$ . In this, the fraction represents $1$ $1$ count out of $6$ $6$ total numbers.

The fraction of blue cars in the picture is $\frac{2}{6}$ $2/6$ . In this, the fraction represents $2$ $2$ numbers out of $6$ $6$ total numbers.

A group of objects may be divided into sub-groups. The number of objects in the sub-group is represented as a fraction of the whole group.

In the given figure:
The Whole (total number of cars): $6$ $6$

Fraction of red cars : $\frac{1}{6}$ $1/6$

Fraction of blue cars : $\frac{2}{6}$ $2/6$

Fraction of Yellow cabs : $\frac{3}{6}$ $3/6$

A fraction is a part of a whole or a part of a group.

Fraction as a part of a group: A fraction represents the number of objects out of a total number of objects.
In this, fraction $\frac{p}{q}$ $p/q$ represents
$p$ $p$ number of items, for every $q$ $q$ number of items in the whole group.

examples

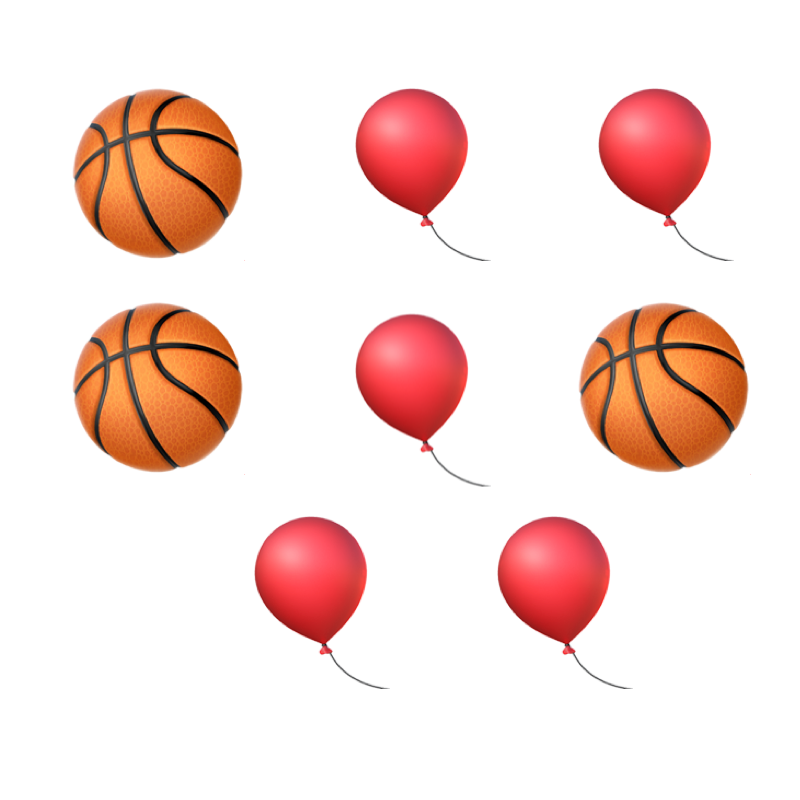
$fraction illustration$

What fraction is basketballs in the group?
The answer is ' $\frac{3}{8}$ $3/8$ '.

What fraction is balloons in the group?
The answer is ' $\frac{5}{8}$ $5/8$ '.

summary

» Fraction of blue cars $\frac{2}{6}$ $2/6$
    → $6$ $6$ cars together make a whole
    → " $2$ $2$ cars" is the given number
    → $\frac{1}{6}$ $1/6$ is the place value

Outline

The outline of material to learn "fractions" is as follows.

• click here for detailed outline of Fractions

→ Part of whole

→ Dividing a group

→ Fractions as Directed numbers

→ Like and Unlike Fractions

→ Proper and Improper Fractions

→ Equivalent & Simplest form

→ Converting unlike and like Fractions

→ Simplest form of a Fraction

→ Comparing Fractions

→ Addition & Subtraction

→ Multiplication

→ Reciprocal

→ Division

→ Numerical Expressions with Fractions

→ PEMA / BOMA