
what you'll learn...
overview
In this page, multiplcation of fractions is explained.
• multiplication in first principles -- repeatedly combining a quantity and measuring the combined and
• simplified procedure : Multiplying numerators and denominators
repeated times
Multiplication of integers or whole numbers:
• is the multiplicand
• is the multiplier
• is the product
Multiplication is repeating the multiplicand the multiplier times -- to get the product.
repeated times =
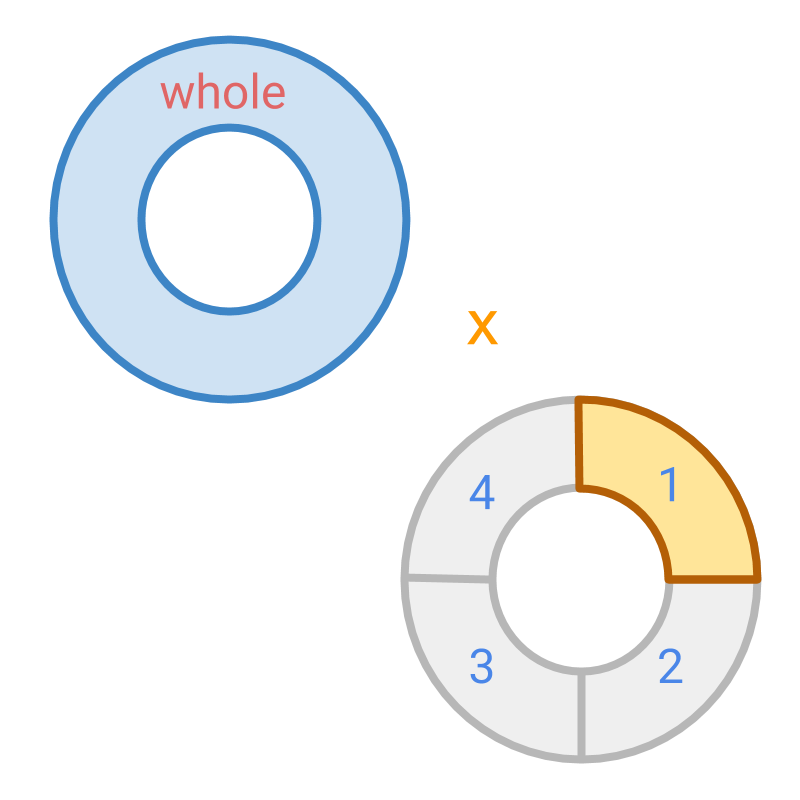
Multiplication by a fraction : Consider
Multiplicand is multiplied by a multiplier .
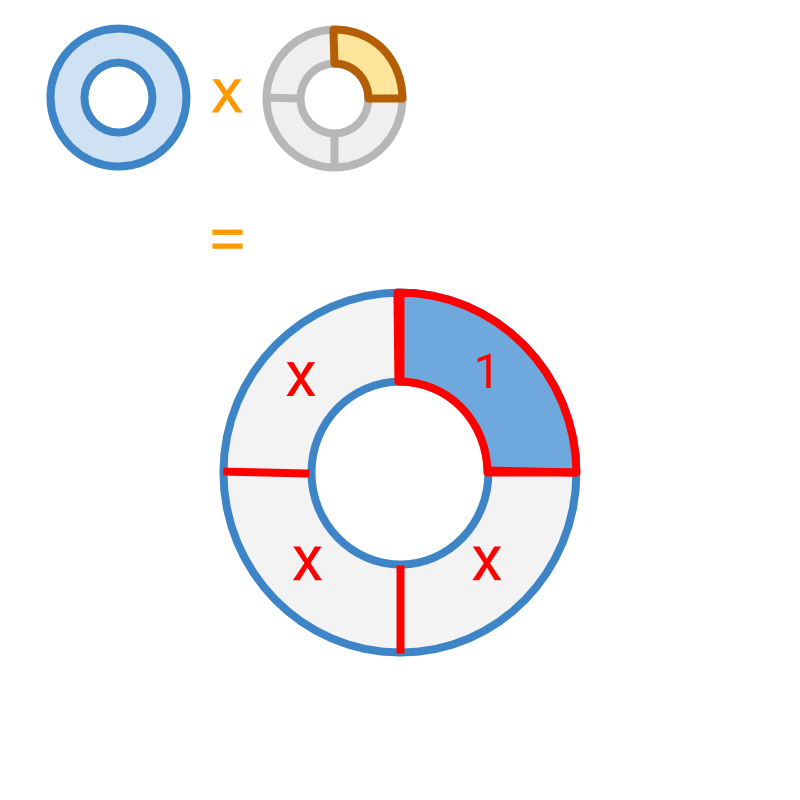
The multiplication is illustrated.
The multiplicand is divided into parts (which is the place value of the multiplier) and product is calculated by selecting part (which is the numerator of the multiplier). The product is .
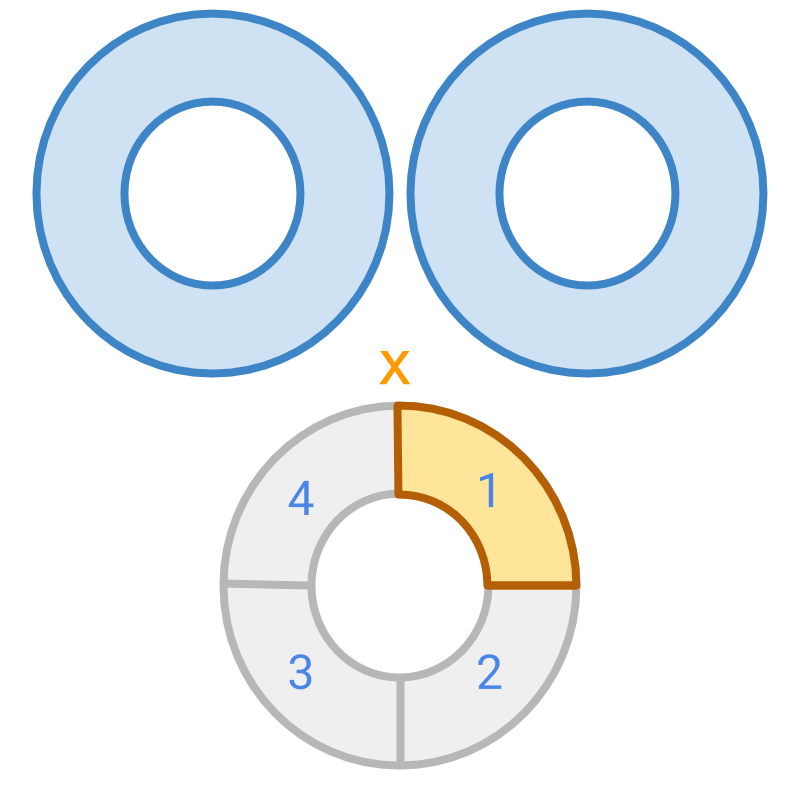
Multiplication by a fraction : Consider
Multiplicand is multiplied by a multiplier .
The multiplication process is illustrated in the next page.

The multiplication is illustrated.
The multiplicand is divided into parts (which is the place value of the multiplier) and product is calculated by selecting part from each (which is the numerator of the multiplier).
The value of one piece with respect to a whole is and pieces are taken. That is, .
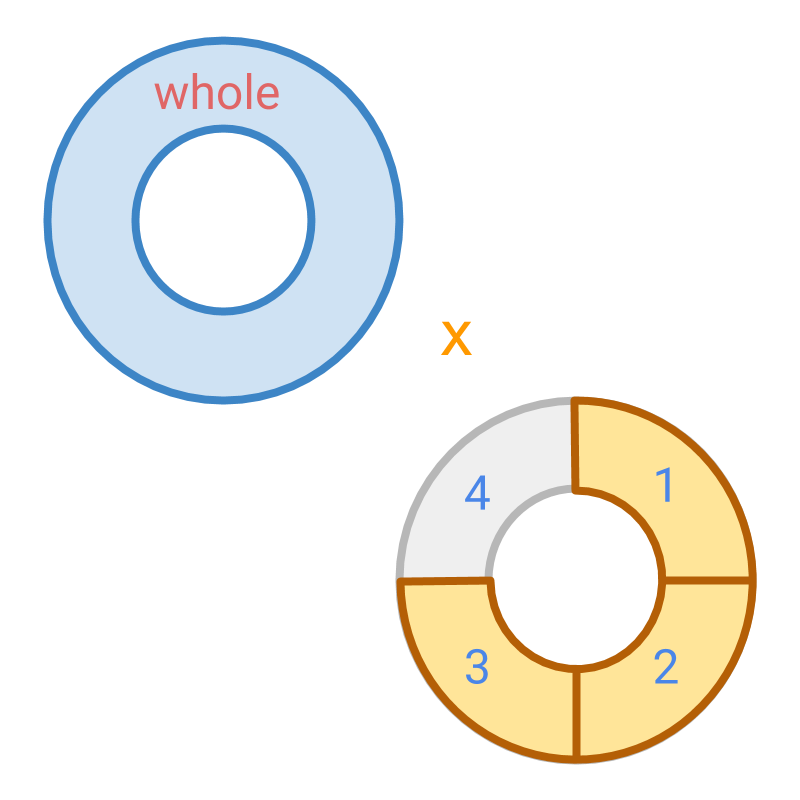
Multiplication by a fraction : Consider
Multiplicand is multiplied by a multiplier .
The multiplication process is illustrated in the next page.

The multiplication is illustrated.
The multiplicand is divided into parts (which is the place value of the multiplier) and product is found by selecting parts (which is the numerator of the multiplier).
The product is pieces in the place value which is .
fraction by fraction
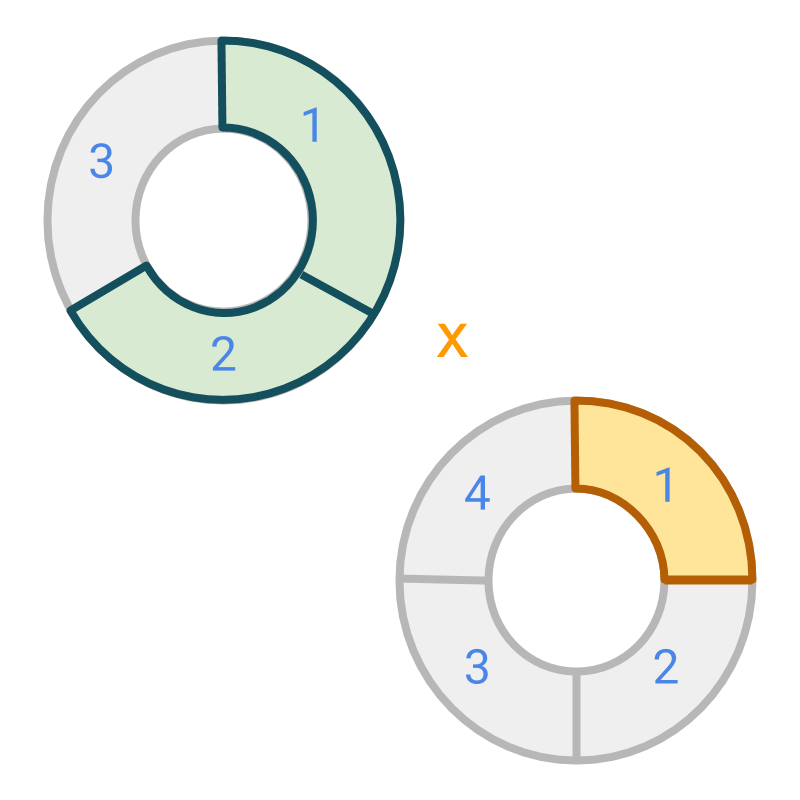
Multiplication by a fraction : consider
Multiplicand is multiplied by a multiplier .
The multiplication process is illustrated in the next page.
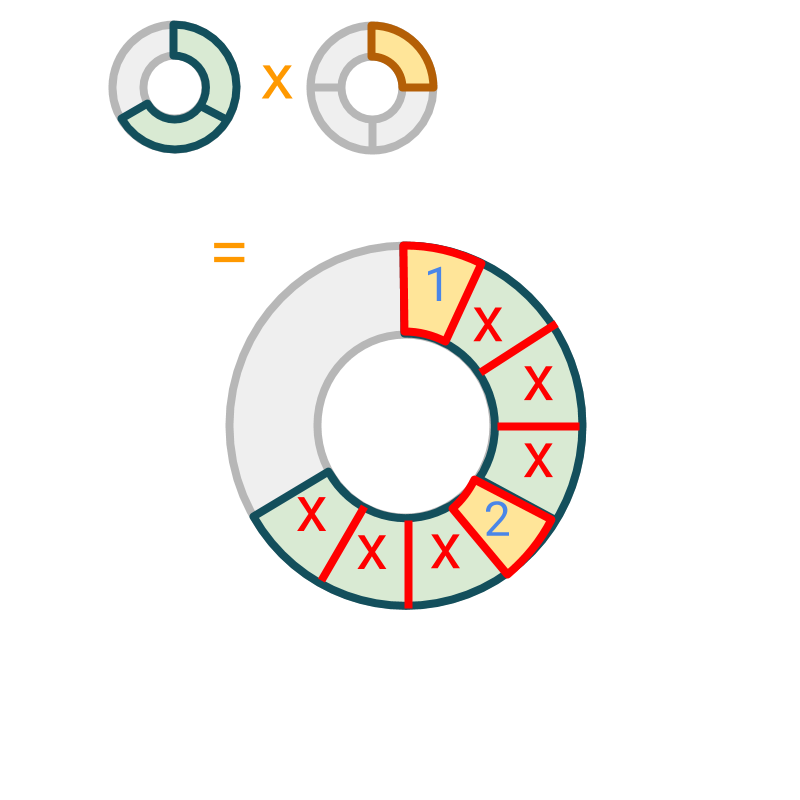
The multiplication is illustrated.
Each part of multiplicand is divided into parts (which is the place value of the multiplier) and product is found by selecting part (which is the numerator of the multiplier) from each.
The product is pieces in the place value which is .
can be simplified to .
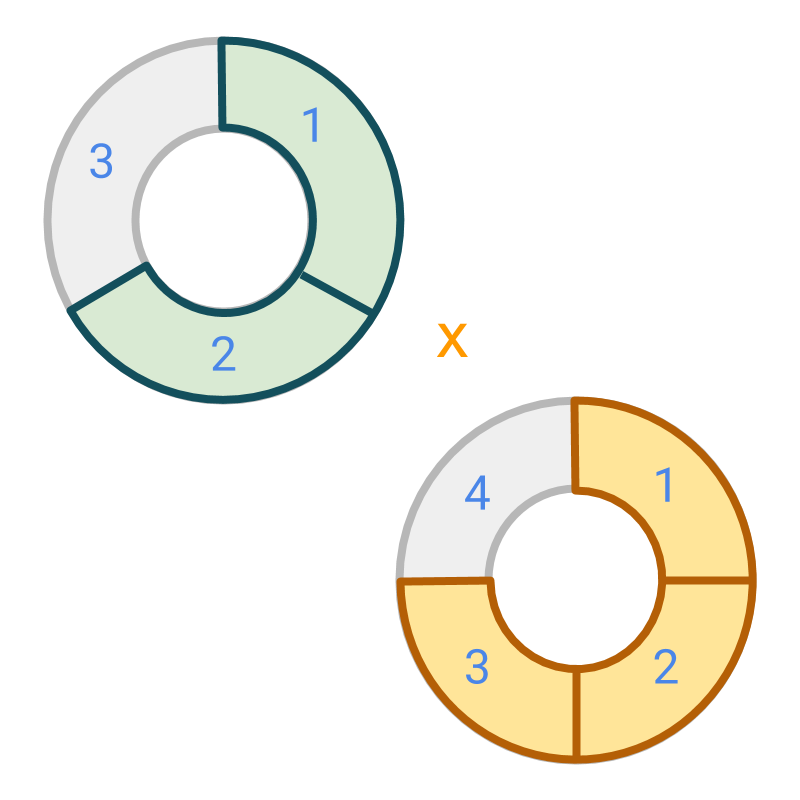
Multiplication by a fraction : Consider
Multiplicand is multiplied by a multiplier .
The multiplication process is illustrated in the next page.
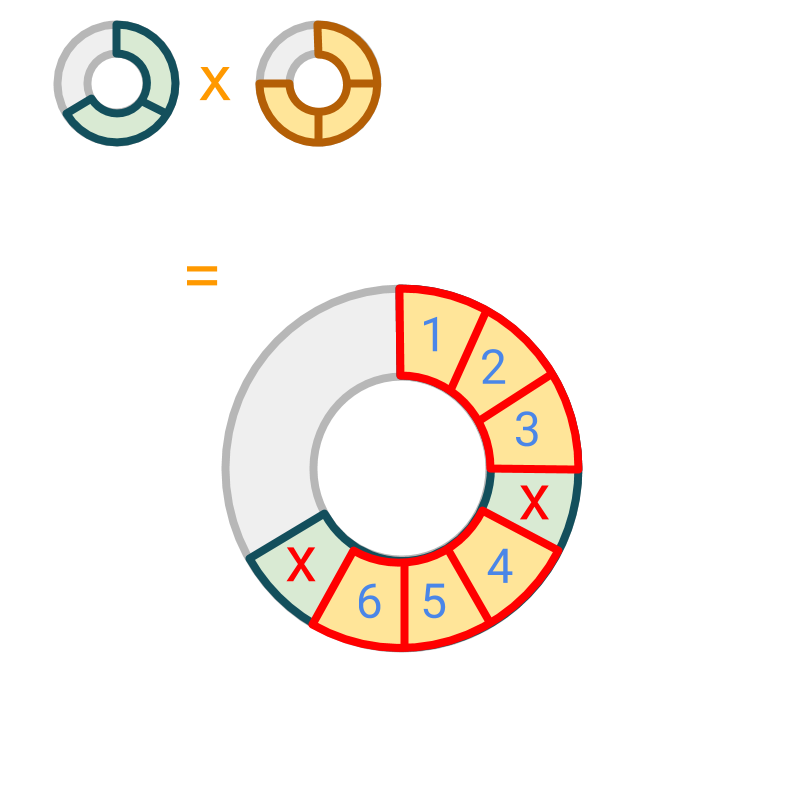
The multiplication is illustrated.
The each part of multiplicand is divided into parts (which is the place value of the multiplier) and product is found by selecting parts (which is the numerator of the multiplier) from each.
The product is pieces in the place value which is .
can be simplified to .
simplify
Having understood the first principles of multiplication of fractions, the procedural simplification for the same is :
• Numerators of multiplicand and multiplier are multiplied to numerator of product.
• Denominators of multiplicand and multiplier are multiplied to denominator of product.
Multiplication is understood in two steps
• The place value of multiplicand is modified by the place value of multiplier.
• The given number of multiplicand is multiplied by the given number of multiplier.
Multiplication of Fractions: For two fractions multiplicand and the multiplier
Every part of multiplicand is split into pieces making it as the modified place value
)
From each of parts of multiplicand parts make the product making parts
parts in place value gives the result
example
Multiply .
The answer is ''
summary
»
»
 → pieces
→ pieces
→ of each of those pieces
→ result is count in place value
→
»
 → pieces in place value
→ pieces in place value
→ of each of those pieces
→ result is count in place value
→
» Procedural Simplification: Multiply numerators and denominators. Cancel any common factors .
→
→
→ common factors and canceled
→
Outline
The outline of material to learn "fractions" is as follows.
• click here for detailed outline of Fractions
→ Part of whole
→ Dividing a group
→ Fractions as Directed numbers
→ Like and Unlike Fractions
→ Proper and Improper Fractions
→ Equivalent & Simplest form
→ Converting unlike and like Fractions
→ Simplest form of a Fraction
→ Comparing Fractions
→ Addition & Subtraction
→ Multiplication
→ Reciprocal
→ Division
→ Numerical Expressions with Fractions
→ PEMA / BOMA