Construction / Practical Geometry (basics) : Construction of Kite

maths > construction-basics

Construction of Kite

what you'll learn...

overview

In this page, constructing kites is explained. It is outlined as follows.

• Properties of kites is explained

• The number of independent parameters in a kite is $1$ $1$

• For a given parameter, construction of kites is approached as combination of triangles (sss, sas, asa, rhs, sal) and using the properties of kites.

Understanding Kite

A kite is a quadrilateral with two pair of equal and adjacent sides.

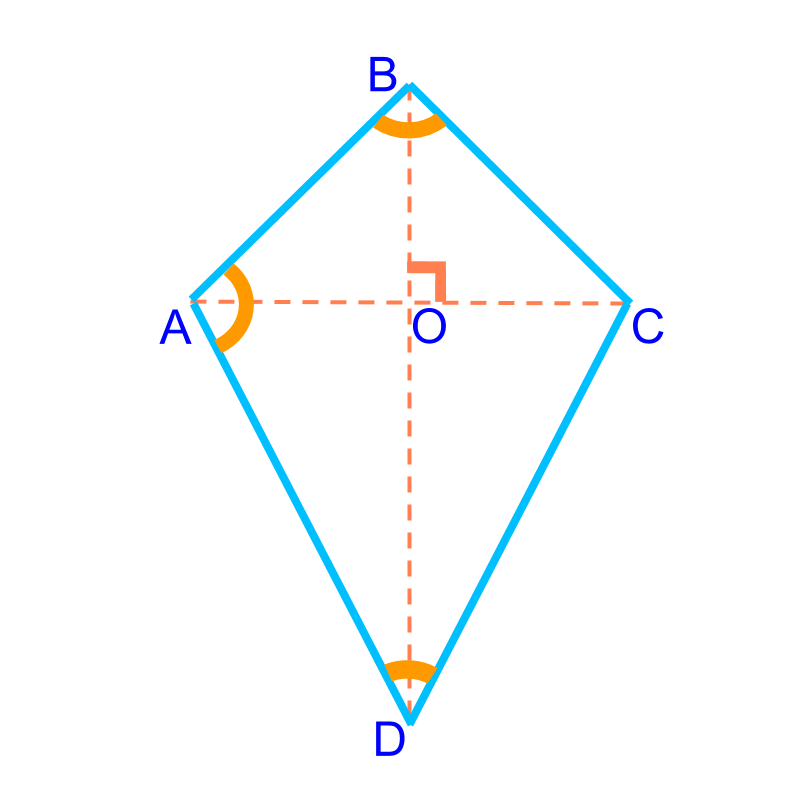
A quadrilateral is defined by $5$ $5$ parameters. For a kite, the following properties provide additional dependency of parameters

• two pair of equal sides

• major diagonal perpendicularly bisects the minor diagonal. the diagonal that divides the kite into two congruent triangles is called major. The other diagonal is the minor diagonal.

• major diagonal bisects the angles at the vertices

• two equal opposite angles and two unequal opposite angles -- all sum up to $360^{\circ}$ $360^@$

A kite is defined by $3$ $3$ parameters.

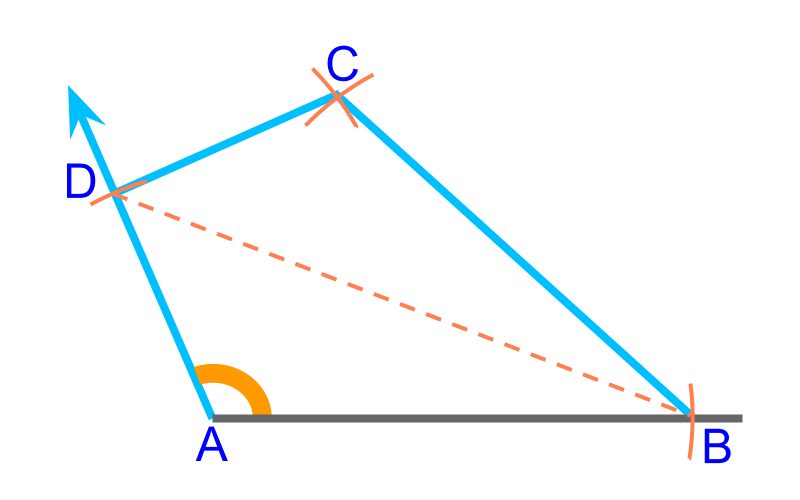
construction of kite with 2 unequal sides, angle

To construct a kite, $2$ $2$ unequal sides ( $¯ ¯¯¯¯ ¯ A B$ $bar(AB)$ , $bar(BC)$ ) and the angle between them ( $/_B$ ) are given. This is illustrated in the figure. To construct, consider this as an SAS triangle in $BAD$ and SSS triangle $BCD$

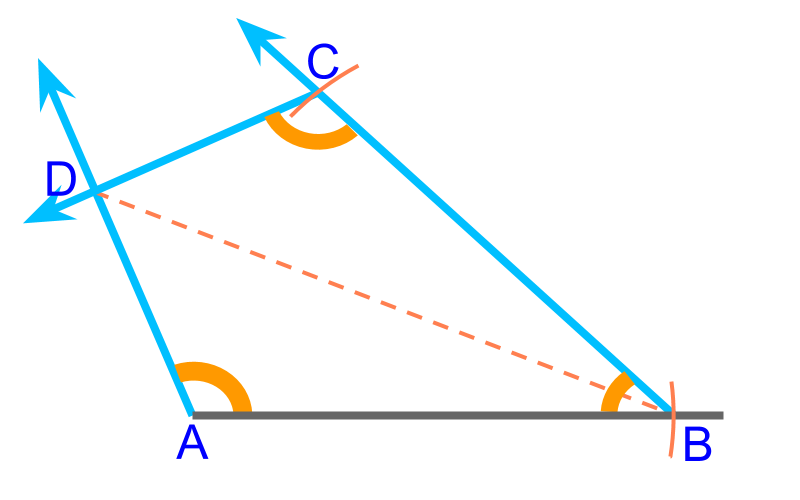
construction of kite with 1 side, and 2 angles

To construct a kite, a side ( $bar(AB)$ ) and $2$ angles ( $/_A$ , $/_B$ ) are given. This is illustrated in the figure. To construct, consider as an SAS triangle in $ABC$ and ASA triangle $ACD$ .

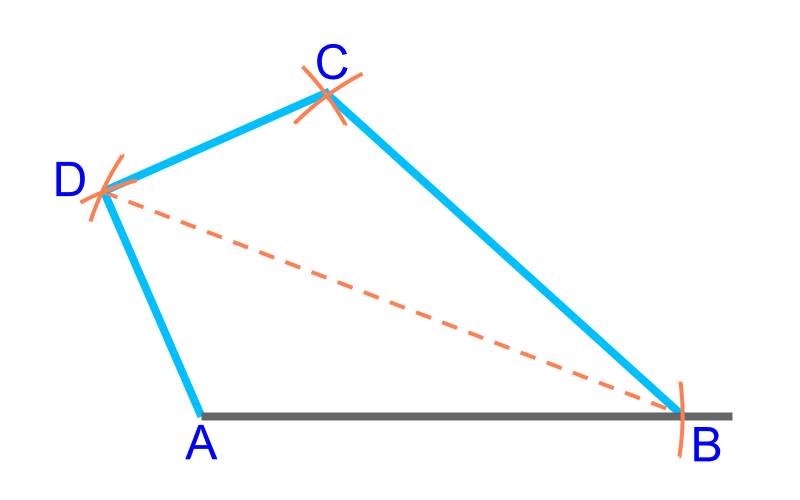
construction of kite with 2 unequal sides, major diagonal

To construct a kite, $2$ unequal sides ( $bar(AB)$ , $bar(BC)$ ) and the major diagonal ( $bar(BD)$ ) are given. This is illustrated in the figure. To construct, consider as two SSS triangles in $ABD$ and $BDC$

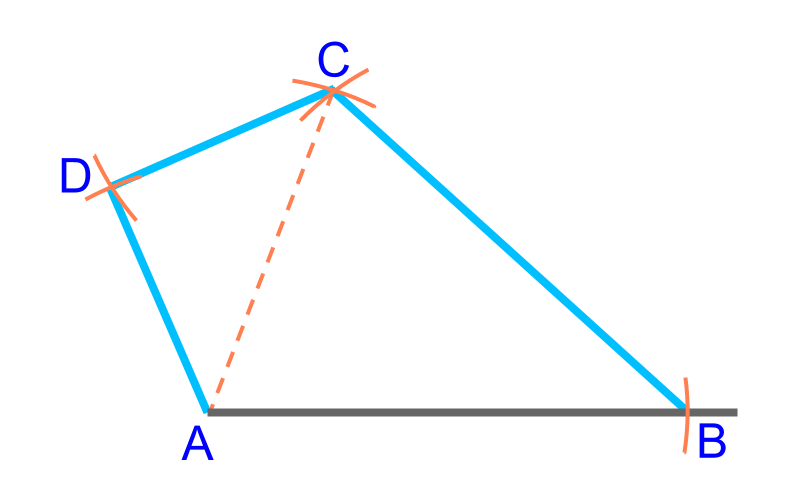
construction of kite with 2 unequal sides, minor diagonal

To construct a kite, $2$ unequal sides ( $bar(AB)$ , $bar(BC)$ ) and the minor diagonal ( $bar(AC)$ ) are given. This is illustrated in the figure. To construct, consider as two isosceles SSS triangles in $ABC$ and $ACD$

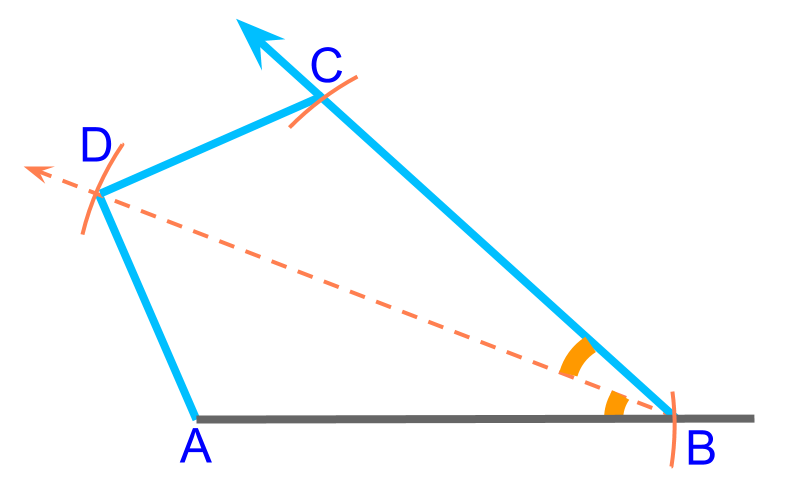
construction of kite with 1 side, major diagonal, angle between them

To construct a kite, a side ( $bar(AB)$ ), the major diagonal ( $bar(BD)$ ), and angle ( $/_ABD$ ) between them are given. This is illustrated in the figure. To construct, consider this as two SAS triangles in $ABD$ and $DBC$

summary

Construction of Kite :

Properties of Kite:

• two pair of equal sides

• major diagonal perpendicularly bisects the minor diagonal. The diagonal that divides the kite as two congruent triangles is the major diagonal.

• major diagonal bisects the angles at the vertices

• two equal opposite angles and two unequal opposite angles sum up to $360^@$

The formulations of questions

• $2$ unequal sides and the angle between them

• $1$ side and $2$ angles

• $2$ unequal sides and the major diagonal

• $2$ unequal sides and the minor diagonal

• $1$ side, major diagonal and the angle between them

Outline