
what you'll learn...
Value of a function
» Value of f(x)f(x)
→ Evaluated at input f(x)∣x=af(x)∣x=a or f(a)f(a)
→ Left-hand-limit limx→a-f(x)limx→a−f(x)
→ Right-hand-limit limx→a+f(x)limx→a+f(x)
» A function f(x)f(x) at x=a is
→ continuous: if f(a) = LHL = RHL
→ defined by value: if f(a) is a real number
→ defined by limit: if f(a)=00 and LHL = RHL
→ not defined: if LHL ≠ RHL and f(a)∉ℝ
Limit of a Function
» If both left-hand-limit and right-hand-limit are equal, it is together referred as "limit of the function"
another motivation
So far, the motivation to examine limits of a function was to evaluate the function at a input value of the argument variable where the function evaluates to 'indeterminate value'.
In this topic, another motivation to examine limits is explained.
Consider f(x)=1x-1 at x=1
by directly substituting x=1
f(1)=11-1=+∞
limx→1+f(x)
=11+δ-1
=1δ
=10=+∞
limx→1-f(x)
=11-δ-1
=1-δ
=-10=-∞.
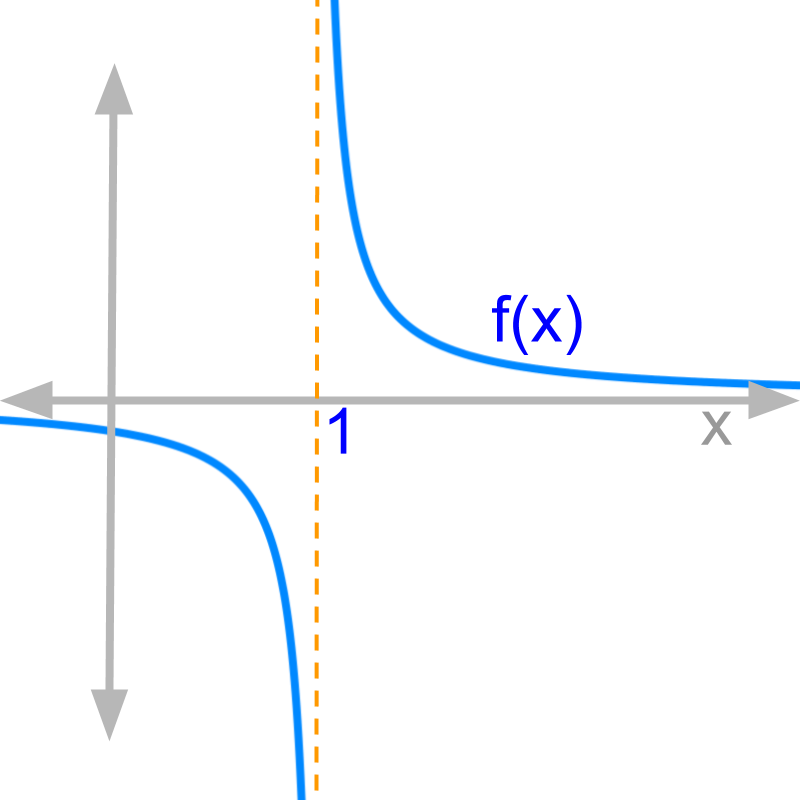
For the function f(x)=1x-1,
•
f(a)=∞
•
limx→1+f(x)=∞
•
limx→1-f(x)=-∞
The plot of the function is given in the figure.
That is, for a value less than x=1, the function is -∞. And at x=1, the function becomes ∞.
The function is not continuous.
continuous
A function f(x) at a given input value x=a is continuous if all the three are equal
f(x)∣x=a
=limx→a-f(x)
=limx→a+f(x)
The word 'continuous' means: unbroken and continue from one side to another without pause in between.
A function is continuous at an input value, if the following three are equal
• function evaluated at the input
• left-hand limit of the function at that input value and
• right-hand limit of the function at that input value.
example
Given function f(x)=2x2, is it continuous at x=0?
The answer is 'Yes, Continuous'. Evaluate the three values of the function and they are equal.
summary
Continuity of a Function: A function f(x) is continuous at x=a if all the following three have a defined value and are equal
• Evaluated at the input value f(x)∣x=a
• left-hand-limit limx→a-f(x)
• right-hand-limit limx-a+f(x)
limit of a function
Given that function f(x) evaluates to indeterminate value at x=a. To evaluate the expected value of f(x)∣x=a, we examine ;
• Left-hand-limit limx→a-f(x)
• Right-hand-limit limx→a+f(x)
If these two limits are equal then the result is referred as "limit of the function at the input value" limx→af(x)
The significance of this is that, most functions have both right-hand-limit and left-hand-limit equal.
summary
Limit of a function: Given function f(x) and that f(x)∣x=a=00.
If limx→a+f(x)=limx→a-f(x),
then the common value is referred as limit of the function limx→af(x).
discontinuous
If a function f(x) is discontinuous at x=a, then what is limx→af(x)?
The answer is 'cannot be computed'. It is given that the function is discontinuous at x=a, and that implies left-hand-limit and right-hand-limits are not equal. In that case, limit of the function cannot be computed without specifying left or right.
summary
Continuity of a Function: A function f(x) is continuous at x=a if all the following three have a defined value and are equal
• Evaluated at the input value f(x)∣x=a
• left-hand-limit limx→a-f(x)
• right-hand-limit limx-a+f(x)
Limit of a function: Given function f(x) and that f(x)∣x=a=00.
If limx→a+f(x)=limx→a-f(x),
then the common value is referred as limit of the function limx→af(x)
Outline
The outline of material to learn "limits (calculus)" is as follows.
Note : click here for detailed outline of Limits(Calculus).
→ Indeterminate and Undefined
→ Indeterminate value in Functions
→ Expected Value
→ Continuity
→ Definition by Limits
→ Geometrical Explanation for Limits
→ Limit with Numerator and Denominator
→ Limits of Ratios - Examples
→ L'hospital Rule
→ Examining a function
→ Algebra of Limits
→ Limit of a Polynomial
→ Limit of Ratio of Zeros
→ Limit of ratio of infinities
→ limit of Binomial
→ Limit of Non-algebraic Functions