Calculus - Limit

maths

Calculus - Limit

Welcome to the only place where the essence of "limit of a function" is explained.

• $\frac{0}{0}$ $0/0$ is called as indeterminate value -- meaning a function evaluating to $\frac{0}{0}$ $0/0$ can take any value, it could be $0$ $0$ , or $1$ $1$ , or $7$ $7$ , or $\infty$ $oo$ , or undefined.

• other forms of indeterminate values are: $\frac{\infty}{\infty}$ $oo/oo$ , $\infty - \infty$ $oo-oo$ , $0^{0}$ $0^0$ , $0 \times \infty$ $0xx oo$ , or $\infty^{0}$ $oo^0$

Rigorous arithmetic calculations may result in $\frac{0}{0}$ $0/0$ , but the expression may take some other value. The objective of limits is to find that value. The details explained are ingenious and found nowhere else.

Once that is explained, the topics in limits are covered.

maths > calculus-limits > numbers-indeterminate-value-undefined-large

Indeterminate value and Undefined Large

The two important numbers "Indeterminate value" and "Undefined large" are explained. It is crucial to understand these two numbers to understand limit of a function. $\frac{0}{0}$ $0/0$ is called as indeterminate value -- meaning a function evaluating to $\frac{0}{0}$ $0/0$ can take any value, it could be $0$ $0$ , or $1$ $1$ , or $7$ $7$ , or $\infty$ $oo$ , or undefined.

Other forms of indeterminate values are: $\frac{\infty}{\infty}$ $oo/oo$ , $\infty - \infty$ $oo-oo$ , $0^{0}$ $0^0$ , $0 \times \infty$ $0xx oo$ , or $\infty^{0}$ $oo^0$

Rigorous arithmetic calculations may result in $\frac{0}{0}$ $0/0$ , but the expression may take some other value. The objective of limits is to find that value.

maths > calculus-limits > indeterminate-value-in-functions

Indeterminate value in functions

Some functions evaluate to indeterminate value at some input values. This is illustrated with an example.

→ eg: $\frac{x^{2} - 4}{x - 2} ∣_{x = 2} = \frac{0}{0}$ $(x^2-4)/(x-2)|_(x=2) = 0/0$ Though the function evaluates to $\frac{0}{0}$ $0/0$ , it may evaluate to a value.

maths > calculus-limits > function-expected-value
Expected Value of a Function

When a function evaluate to indeterminate value at $x = a$ $x=a$ , Considering functions in abstraction, the value at $x = a$ $x=a$ can be assumed to be that of $x = a \pm δ$ $x=a+- delta$ with $δ$ $delta$ close to $0$ $0$ . This, is formuated into two limits, left-hand-limit and right-hand-limit are introduced and explained.

maths > calculus-limits > continuity-limits
Continuity of a Function at an input value

Even when functions have a defined value at an input value, the function may be discontinuous. Limit of the function at that input value can be used to determine continuity.

» A function $f (x)$ $f(x)$ at $x = a$ $x=a$ is
    → continuous: if $f (a)$ $f(a)$ = LHL = RHL

maths > calculus-limits > function-defined-limits
Function defined at an input value

The two conditions, to figure out if a function is defined at an input value or not, are explained.

» A function $f (x)$ $f(x)$ at $x = a$ $x=a$ is
    → defined by value: if $f (a)$ $f(a)$ is a real number

maths > calculus-limits > understanding-limits-geometrical
Understanding limits with the graph of the function

Geometrical meaning of finding limit of a function is explained.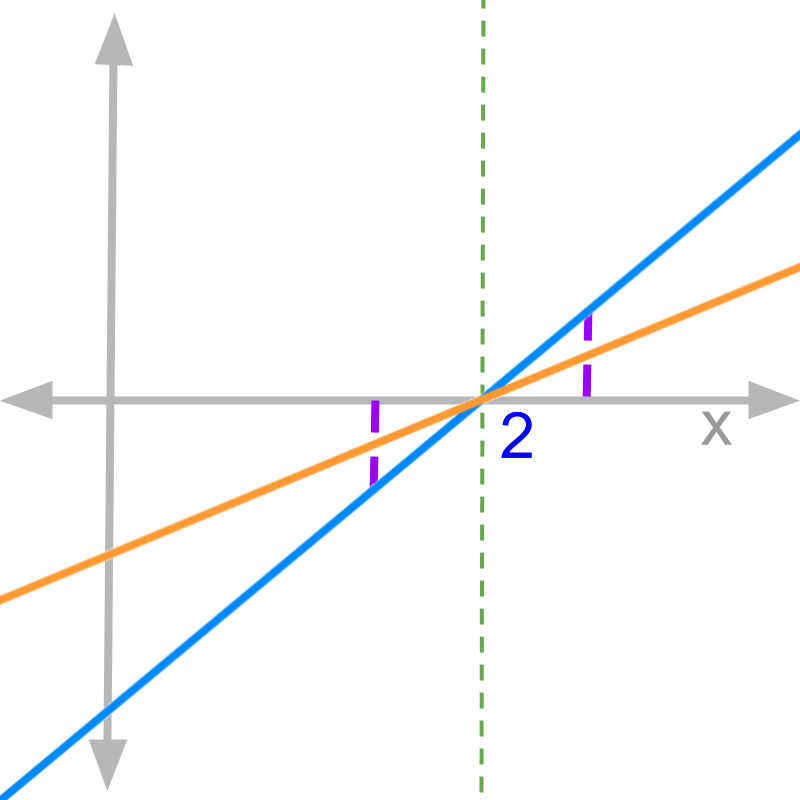

maths > calculus-limits > limits-of-numerator-denominator
Understanding limits with Graphs of Numerator and Denominator

The function for which limit is computed is considered as two constituent functions of numerator and denominator. The limit of the function is explained with the graphs of numerator and denominator.

Slopes of numerator and denominator at the point $x = a$ $x=a$ decide the limit of $f (x)$ $f(x)$ at $x = a$ $x=a$ . The limit of the function is computed as slope of numerator divided by slope of denominator, under some conditions.

maths > calculus-limits > limits-of-numerator-denominator-examples
Understanding Limits : Examples

More examples are explained to evaluate limit of a function, using the graphs of the numerator and denominator. Slopes of numerator and denominator at the point $x = a$ $x=a$ decide the limit of $f (x)$ $f(x)$ at $x = a$ $x=a$ .

The limit of the function is computed as slope of numerator divided by slope of denominator, under some conditions.

maths > calculus-limits > limits-l-hospital-rule
L'Hospital's Rule

The function, for which limit is computed, is considered as two constituent functions of numerator and denominator. To find the limit of the function, differentiate the numerator and denominator.

maths > calculus-limits > examining-function-at-input-value
Examining Function at an input value

Let us first Revise the basics learned in earlier lesson and summarizing the understanding. Then the following are explained

" Limit of a Continuous Function

" Limit of a defined Function

" Limit of Piecewise function

" Limit of Function with abrupt change

" Limit of Functions that are not defined

maths > calculus-limits > algebra-of-limits
Algebra of Limits

This topic explains the observations one has to take before applying algebra of limits.

» Finding limit of function as sub-expressions

    → $f (x) \pm g (x)$ $f(x) +- g(x)$

    → $f (x) \times g (x)$ $f(x) xx g(x)$

    → $f (x) \div g (x)$ $f(x) -: g(x)$

    → ${[f (x)]}^{n}$ $[f(x)]^n$

    → $f (x)$ $f(x)$ and $y = g (x)$ $y=g(x)$

maths > calculus-limits > limit-of-polynomials
Limit of Polynomials

Finding limit of standard polynomial expressions is explained with an example.

Apply Algebra of Limits
For a function $f (x) = a_{n} x^{n}$ $f(x) = a_nx^n$ $+ a_{n - 1} x^{n - 1}$ $+a_(n-1)x^(n-1)$ $+ \dots$ $+ cdots$ $+ a_{1} x^{1}$ $+ a_1x^1$ $+ a_{0}$ $+ a_0$

$lim_{x \to a} f (x)$ $lim_(x->a) f(x)$

$= a_{n} lim_{x \to a} x^{n}$ $quad quad = a_n lim_(x->a) x^n$ $+ a_{n - 1} lim_{x \to a} x^{n - 1}$ $+a_(n-1) lim_(x->a) x^(n-1)$ $+ \dots$ $+ cdots$ $+ a_{1} lim_{x \to a} x^{1}$ $+ a_1 lim_(x->a) x^1$ $+ a_{0}$ $+ a_0$

maths > calculus-limits > limit-ratio-of-zero
Limit of Ratios

Finding limit of standard ratios evaluating to $\frac{0}{0}$ $0/0$ is explained with examples.

» for defined polynomials, algebra of limits apply.

» For polynomials evaluating to $\frac{0}{0}$ $0/0$ value
    → factorize numerator and denominator

    → cancel common factors

    → organize to standard results

    → apply limit to modified expressions

maths > calculus-limits > limit-ratio-of-infinity
Limit of functions evaluating to $\infty$ $oo$

Finding limit of standard ratios evaluating to $\frac{\infty}{\infty}$ $oo/oo$ or $\infty - \infty$ $oo-oo$ is explained with examples.

» Organize the sub-expressions to the following

    → $\frac{a}{\infty} = 0$ $a/oo = 0$

    → $\infty \pm a = \infty$ $oo+-a = oo$

    → $\infty \times a = \infty$ $oo xx a = oo$ when $a \neq 0$ $a != 0$

    → $\infty \times \infty = \infty$ $oo xx oo = oo$

    → $\infty^{n} = \infty$ $oo^n = oo$ when $n \neq 0$ $n != 0$

    → $lim_{x \to \infty} \frac{x}{x} = 1$ $lim_(x->oo) x/x =1$

    → $lim_{x \to - \infty} \frac{x}{x} = 1$ $lim_(x->-oo) x/x =1$

    → $lim_{x \to \infty} a^{x}$ $lim_(x->oo) a^x$ `=0 text( if ) 0 $= \infty if a > 1$ $quad quad quad = oo text( if ) a>1$

maths > calculus-limits > limit-of-binomial
Limits involving Binomial Expressions

Factoring binomials is revised and the limit involving binomials is explained with an example.

Limits involving Binomial Expressions
» Special case of canceling factors in numerator and denominator
    → $lim_{x \to a} \frac{x^{n} - a^{n}}{x - a} = n a^{n - 1}$ $lim_(x->a)(x^n-a^n)/(x-a) = na^(n-1)$

» With change of variable $x = 1 + y$ $x=1+y$ and constant $a = 1$ $a=1$
    → $lim_{y \to 0} \frac{{(1 + y)}^{n} - 1}{y} = n$ $lim_(y->0) ((1+y)^n - 1)/y = n$

maths > calculus-limits > limit-non-algebraic-functions
Limit of Trigonometric / Logarithmic / Exponential Functions

Standard limits involving trigonometric function are explained.