Trigonometry (advanced) : Trigonometric values for Any Angle: First Principles

maths > advanced-trigonometry

Trigonometric values for Any Angle: First Principles

what you'll learn...

Trigonometric Values : First Principles

» The angle $ω$ $omega$ is measured from positive x-axis
    → x and y coordinates takes sign.
Quickly follow the sign of x and y projections to find sign of trigonometric values. No need to memorize. 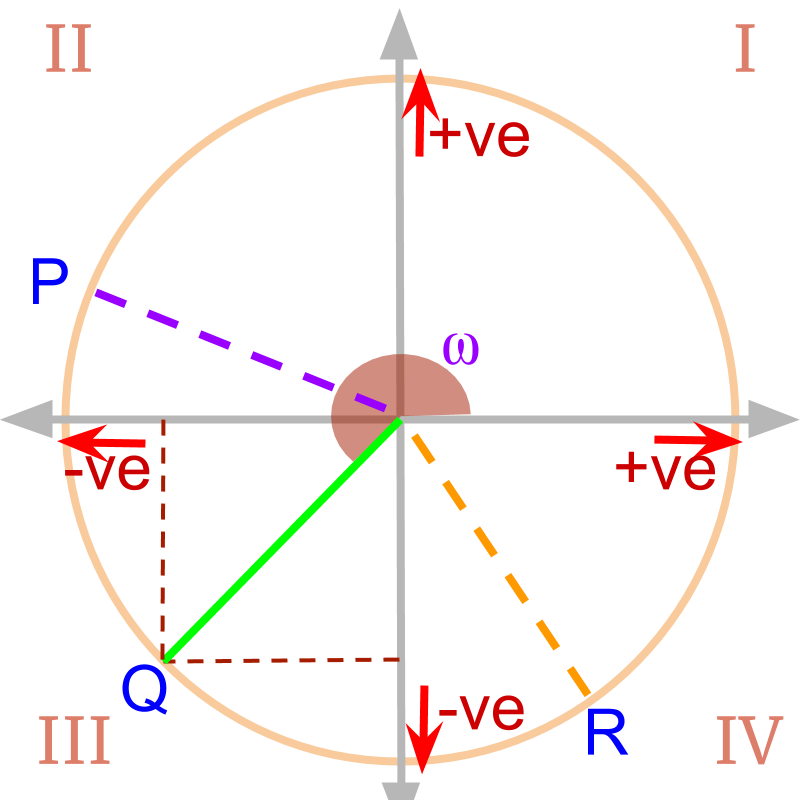trigonometry reference angles » $P$ $P$ is in the second quadrant
    → -ve x projection
    → +ve y projection

» $Q$ $Q$ is in third quadrant
    → -ve: both x and y projections

» $R$ $R$ is in fourth quadrant
    → +ve x projection
    → -ve y projection

second quadrant

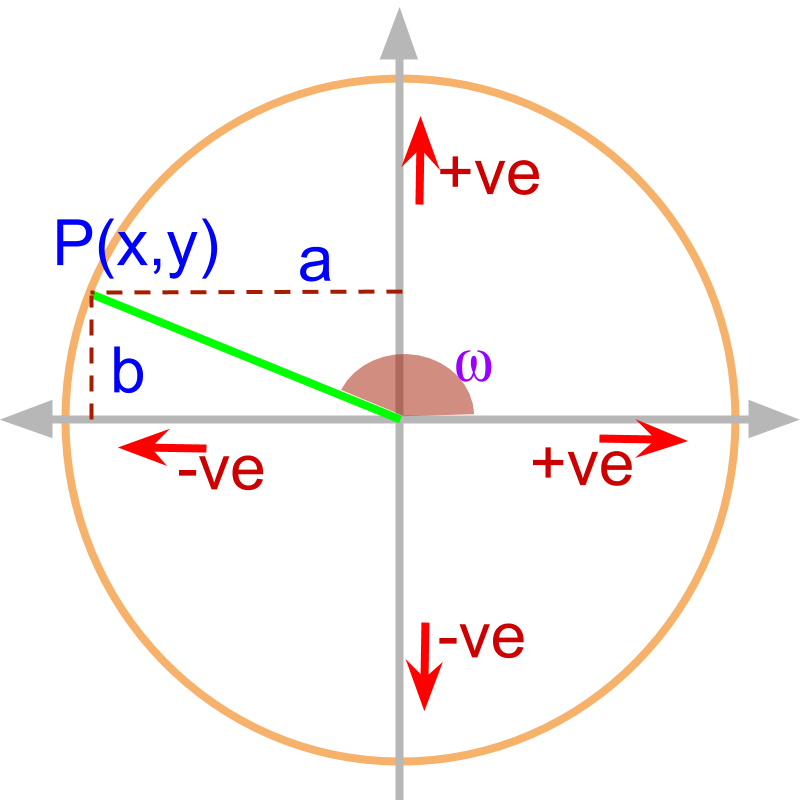
The angle $ω$ $omega$ is shown in the figure. The magnitude of projections on x axis and y axis is given as $a$ $a$ and $b$ $b$ .

It is noted that the projection along $x -$ $x-$ axis is $- a$ $-a$ and so

$\tan θ = \frac{b}{- a}$ $tan theta = b/(-a)$ .

The trigonometric ratios are computed from the x and y axis projections.
Note that the projections are given for point on unit circle. so $\sqrt{a^{2} + b^{2}} = 1$ $sqrt(a^2 + b^2) = 1$ .

$\sin ω = \frac{b}{1}$ $sin omega = b/1$

$\cos ω = \frac{- a}{1}$ $cos omega = (-a)/1$

$\tan ω = \frac{b}{- a}$ $tan omega = b/(-a)$

third quadrant

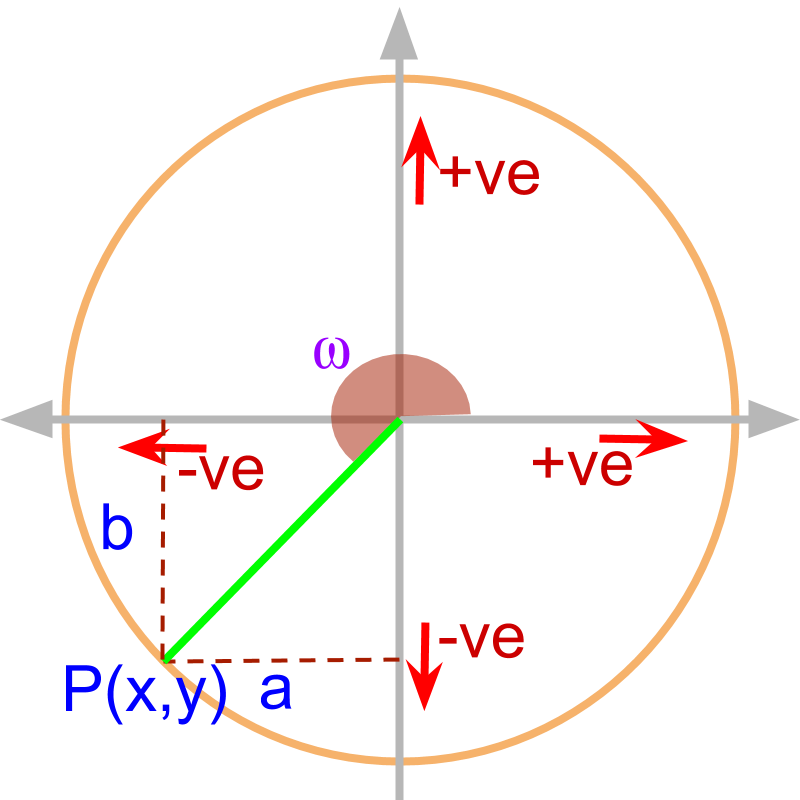
The angle $ω$ $omega$ is shown in the figure. The magnitude of projections on x axis and y axis is given as a and b.

Considering the projections along x axis and y axis as $- a$ $-a$ and $- b$ $-b$ ,

$\tan ω = \frac{- b}{- a}$ $tan omega = (-b)/(-a)$

Note that the sign of the numerator and denominator provide information as to if the angle is in first quadrant or 3rd quadrant.

The trigonometric ratios are computed from the x and y axis projections.
Note that the projections are given for point on unit circle. so $\sqrt{a^{2} + b^{2}} = 1$ $sqrt(a^2 + b^2) = 1$ .

$\sin ω = \frac{- b}{1}$ $sin omega = (-b)/1$

$\cos ω = \frac{- a}{1}$ $cos omega = (-a)/1$

$\tan ω = \frac{- b}{- a}$ $tan omega = (-b)/(-a)$

third quadrant

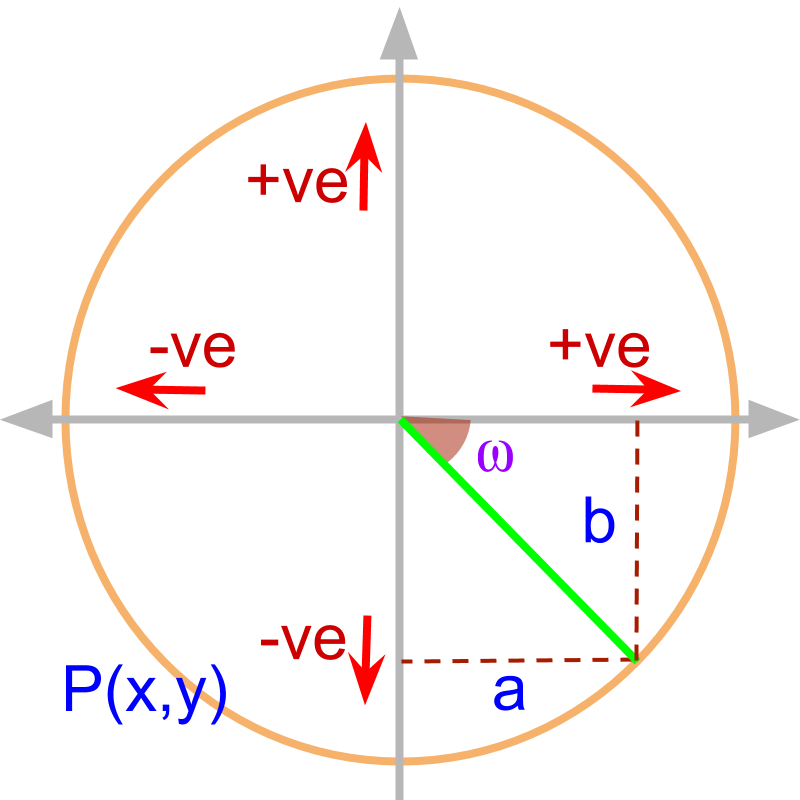
The angle $ω$ $omega$ is negative and is shown in the figure. The magnitude of projections on x axis and y axis is given as a and b.

Considering the projections on x axis and y axis as $a$ $a$ and $- b$ $-b$ , $\tan ω = \frac{- b}{a}$ $tan omega = (-b)/a$

The trigonometric ratios are computed from the x and y axis projections.
Note that the projections are given for point on unit circle. so $\sqrt{a^{2} + b^{2}} = 1$ $sqrt(a^2 + b^2) = 1$ .

$\sin ω = \frac{- b}{1}$ $sin omega = (-b)/1$

$\cos ω = \frac{a}{1}$ $cos omega = a/1$

$\tan ω = \frac{- b}{a}$ $tan omega = (-b)/a$

large angle

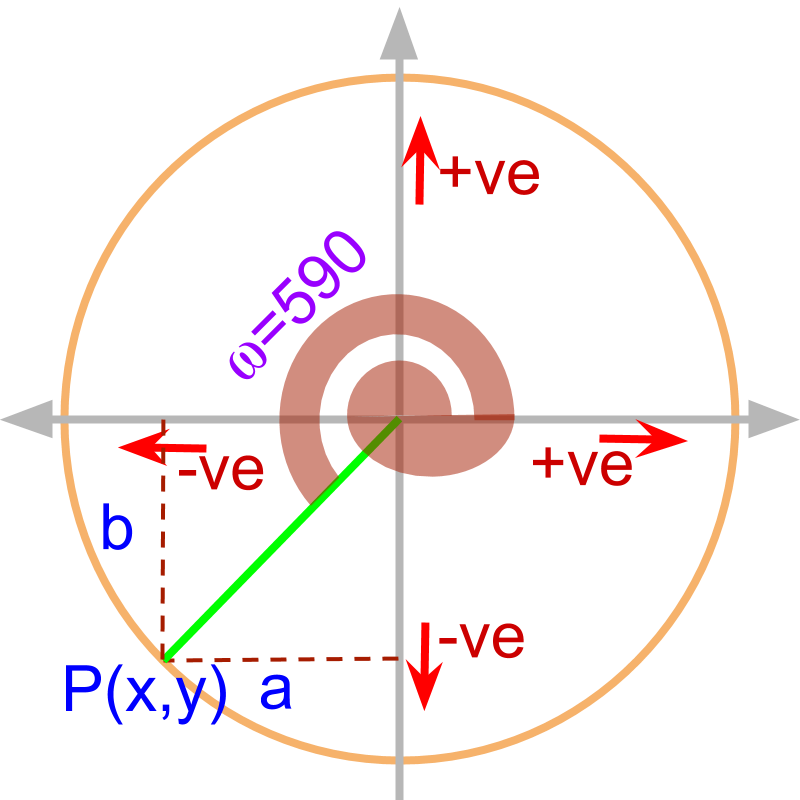
The angle omega equals $590^{\circ}$ $590^@$ is shown in the figure. The magnitude of projections on x axis and y axis is given as a and b.

${\tan 590}^{\circ} = \frac{- b}{- a}$ $tan 590^@ = (-b)/(-a)$

The trigonometric ratios are computed from the x and y axis projections.

$\sin ω = \frac{- b}{1}$ $sin omega = (-b)/1$

$\cos ω = \frac{- a}{1}$ $cos omega = (-a)/1$

$\tan ω = \frac{- b}{- a}$ $tan omega = (-b)/(-a)$

For any angle the trigonometric values are computed using the projections of point on unit circle at the given angle on to x and y axis.

summary

First Principles to find Trigonometric Ratios for any Angle: For the given angle, find the projections of point on unit circle at the given angle on to x and y axes. The projections are signed values. The trigonometric ratios are computed as

• $\sin ω = y projection$ $sin omega = y text( projection)$

• $\cos ω = x projection$ $cos omega = x text( projection)$

• $\tan ω = \frac{y projection}{x projection}$ $tan omega = (y text( projection))/(x text( projection))$

Outline

It is advised to do the firmfunda version of "basics of Trigonometry" course before doing this.

The outline of material to learn "Advanced Trigonometry" is as follows.
Note: go to detailed outline of Advanced Trigonometry

→ Unit Circle form of Trigonmetric Values

→ Trigonometric Values in all Quadrants

→ Trigonometric Values or any Angles : First Principles

→ Understanding Trigonometric Values in First Quadrant

→ Trigonometric Values in First Quadrant

→ Trigonometric Values of Compound Angles: Geometrical Proof

→ Trigonometric Values of Compound Angles: Algebraic Proof

→ Trigonometric Values of Compound Angles: tan cot

→ Trigonometric Values of Compound Angles: more results