Integral Calculus

maths

Integral Calculus

Integration takes a new name "continuous aggregate". That is, a quantity, given as a function, is aggregated continuously over an interval.The details explained are ingenious and provided nowhere else.

Learn in this:

• First Principles of Integration : Continuous aggregate

• Graphical Meaning of Integration : Area under a curve

• Definition of definite and indefinite integrals

• Fundamental theorem of Calculus : Integration as anti-derivatives

Apart from these, the algebra of integrals and various results of integration of standard functions are explained.

maths > integral-calculus > integration-application-scenario

Integral Calculus: Understanding Application Scenarios

In this page, the application scenario of integrals is explained with examples. We consider pair of related quantities. One quantity is the cause and the other is the effect. These are related by a function involving continuous aggregate.

maths > integral-calculus > integration-first-principles

Integration: First Principles

In this page, integration is defined in first principles.

Integration or integral of a function

In an interval from $0$ $0$ to $x$ $x$ , the continuous aggregate of function $f (x)$ $f(x)$ is the integral of the function.

$\int f (x) d x$ $int f(x) dx$

$= c + \int_{0}^{x} f (x) d x$ $= c + int_0^x f(x)dx$

$= c + lim_{n \to \infty} \sum_{i = 1}^{n} f (\frac{i x}{n}) \frac{x}{n}$ $= c + lim_(n->oo) sum_(i=1)^n f((i x)/n) x/n$

maths > integral-calculus > integration-graphical-meaning

Integration: Graphical Meaning

In this page, graphical meaning of integration is discussed with examples.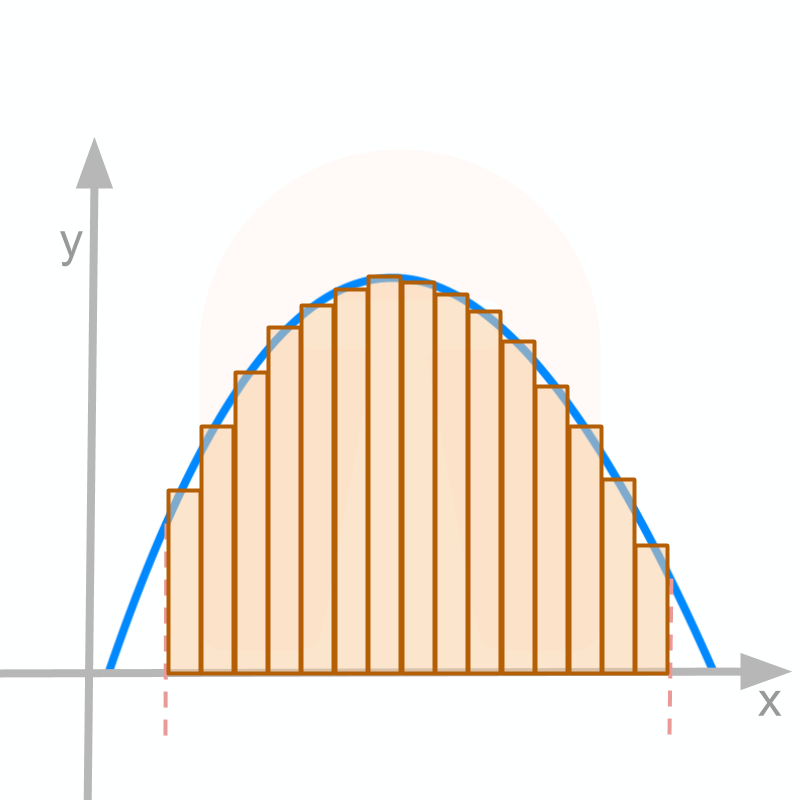 graphical meaning of integral

maths > integral-calculus > definitions-of-integrals

Defining integral in different forms

In this page, indefinite integral and definite integrals are explained. In definite integrals, various forms of limit of summation are introduced. » Indefinite Integral
    → $\int f (x) d x$ $int f(x)dx$
    → a function of a variable
    → anti-derivative

» Definite Integral
    → $\int_{a}^{b} f (x) d x$ $int_a^b f(x)dx$
    → a numerical value
    → area under the curve between $x = a$ $x=a$ and $x = b$ $x=b$

maths > integral-calculus > integration-fundamental-theorem-of-calculus

Fundamental Theorem of Calculus

In this page, the fundamental theorem of calculus is stated and proven. Anti-derivatives are introduced as the function that when differentiated, gives the integrand.

Fundamental Theorem of Calculus :

For functions $f (x), h (x), g (x)$ $f(x), h(x), g(x)$ as in
$h (x) = \frac{d}{d x} f (x)$ $h(x) = d/(dx) f(x)$
$f (x) = \int_{a}^{x} g (x) d x$ $f(x) = int_a^x g(x)dx$

    → First part states that $h (x) = g (x)$ $h(x) = g(x)$ ; that is $\frac{d}{d x} \int_{a}^{x} g (x) d x = g (x)$ $d/(dx) int_a^x g(x) dx = g(x)$
derivative of integral of a function is the function

    → First part implies that to find $f (x) = \int_{a}^{x} g (x) d x$ $f(x) = int_a^x g(x)dx$ , find the inverse relationship $\frac{d}{d x} f (x) = g (x)$ $d/(dx) f(x) = g(x)$ and thus, anti-derivative is defined.
integral of a function is anti-derivative of the function

    → Second part states that $\int_{a}^{b} h (x) d x = f (b) - f (a)$ $int_a^b h(x) dx = f(b)-f(a)$
That is $\int_{a}^{b} \frac{d}{d x} f (x) d x = f (b) - f (a)$ $int_a^b d/(dx) f(x) dx = f(b)-f(a)$ .
definite integral of derivative of a function is the difference of function evaluated at the limits

    → Since the second part is true for any value $x$ $x$ in the interval $[a, b]$ $[a,b]$ , it implies that $\int_{a}^{x} \frac{d}{d x} f (x) d x = f (x) + c$ $int_a^x d/(dx) f(x) dx = f(x) +c$ .
indefinite integral of derivative of a function is the function plus an initial value

maths > integral-calculus > integration-algebra-of-integrals

Understanding Algebra of Integration

In this page, what is algebra of integrals and conditions under which it is applicable are discussed. The integrals of functions that are given as arithmetic operation of multiple functions is discussed. The arithmetic operations are multiplication by a constant, addition, and subtraction. The properties of definite integrals, specifically based on the limits of integration, are explained.

» Indefinite integrals under Basic Arithmetic Operations
    → $\int a u d x = a \int u d x$ $int audx = a int udx$
    → $\int (u + v) d x = \int u d x + \int v d x$ $int (u+v)dx = int udx + int vdx$
    → $\int (u - v) d x = \int u d x - \int v d x$ $int (u-v)dx = int udx - int vdx$

» Properties of definite integrals

    → $\int_{a}^{b} f (x) d x = \int_{a}^{b} f (y) d y$ $int_a^b f(x) dx = int_a^b f(y) dy$

    → $\int_{a}^{a} f (x) d x = 0$ $int_a^a f(x) dx = 0$

    → $\int_{a}^{b} f (x) d x = - \int_{b}^{a} f (x) d x$ $int_a^b f(x) dx = -int_b^a f(x)dx$

    → $\int_{a}^{b} f (x) d x$ $int_a^b f(x)dx$ $+ \int_{b}^{c} f (x) d x =$ $+ int_b^c f(x)dx=$ $\int_{a}^{c} f (x) d x$ $int_a^c f(x)dx$

maths > integral-calculus > integral-antiderivatives-standard-results

Standard Results of Anti-Derivatives

In this page, the standard results of derivatives are revised and the same is given in anti-derivative form.

» Indefinite integrals under Basic Arithmetic Operations
    → $\int a u d x = a \int u d x$ $int audx = a int udx$
    → $\int (u + v) d x = \int u d x + \int v d x$ $int (u+v)dx = int udx + int vdx$
    → $\int (u - v) d x = \int u d x - \int v d x$ $int (u-v)dx = int udx - int vdx$

» Properties of definite integrals

    → $\int_{a}^{b} f (x) d x = \int_{a}^{b} f (y) d y$ $int_a^b f(x) dx = int_a^b f(y) dy$

    → $\int_{a}^{a} f (x) d x = 0$ $int_a^a f(x) dx = 0$

    → $\int_{a}^{b} f (x) d x = - \int_{b}^{a} f (x) d x$ $int_a^b f(x) dx = -int_b^a f(x)dx$

    → $\int_{a}^{b} f (x) d x$ $int_a^b f(x)dx$ $+ \int_{b}^{c} f (x) d x =$ $+ int_b^c f(x)dx=$ $\int_{a}^{c} f (x) d x$ $int_a^c f(x)dx$

maths > integral-calculus > integration-understanding-complexity-and-methods

Methods of Integration

In this page, the standard forms of integration with multiplication and division are explained. Multiplication or division in integrand can be converted into something for which integration can be worked out.

» Indefinite integrals under Basic Arithmetic Operations
    → $\int a u d x = a \int u d x$ $int audx = a int udx$
    → $\int (u + v) d x = \int u d x + \int v d x$ $int (u+v)dx = int udx + int vdx$
    → $\int (u - v) d x = \int u d x - \int v d x$ $int (u-v)dx = int udx - int vdx$

» Properties of definite integrals

    → $\int_{a}^{b} f (x) d x = \int_{a}^{b} f (y) d y$ $int_a^b f(x) dx = int_a^b f(y) dy$

    → $\int_{a}^{a} f (x) d x = 0$ $int_a^a f(x) dx = 0$

    → $\int_{a}^{b} f (x) d x = - \int_{b}^{a} f (x) d x$ $int_a^b f(x) dx = -int_b^a f(x)dx$

    → $\int_{a}^{b} f (x) d x$ $int_a^b f(x)dx$ $+ \int_{b}^{c} f (x) d x =$ $+ int_b^c f(x)dx=$ $\int_{a}^{c} f (x) d x$ $int_a^c f(x)dx$

maths > integral-calculus > integration-by-substitution

Integration by Substitution

In this page, integration by substitution is explained with examples.

Integration by Substitution :

When $f (x) = h (g (x)) g' (x)$ $f(x) = h(g(x)) g′(x)$

then,
$\int f (x) d x = \int h (y) d y$ $int f(x)dx = int h(y)dy$
where $y = g (x)$ $y=g(x)$
and $d y = g' (x) d x$ $dy = g′(x)dx$ and so
» $\int f (x) d x$ $int f(x)dx$ $= [\int h (y) d y] ∣_{y = g (x)} + c$ $= [int h(y)dy]|_(y=g(x)) + c$

maths > integral-calculus > integration-using-identities

Integration using Identities

In this page, integration using identities is explained with examples.

Integration using Identities :

when $f (x) = Π_{i = 1}^{n} g_{i} (x)$ $f(x) = Pi_(i=1)^n g_i(x)$ , use identities to convert to $f (x) = \sum_{i = 1}^{m} h_{i} (x)$ $f(x) = sum_(i=1)^m h_i(x)$ , such that integration can be individually performed on $h_{i} (x)$ $h_i(x)$

maths > integral-calculus > integration-by-parts

Integration by Parts

In this page, integration by parts is explained with examples.

Integration by Parts :

» $\int f (x) g (x) d x$ $int f(x) g(x) dx$ $= f (x) \int g (x) d x$ $= f(x) intg(x)dx$ $- \int [\int g (x) d x] [\frac{d}{d x} f (x)] d x$ $- int [int g(x) dx] [d/(dx)f(x)] dx$

→ $\int e^{x} (h (x) + h' (x)) d x$ $int e^x (h(x)+h′(x)) dx$ $= e^{x} h (x) + c$ $=e^x h(x) + c$

maths > integral-calculus > integration-by-partial-fraction

Integration by Partial Fraction

In this page, Integration by Partial Fraction is explained with examples.

Integration by Partial Fraction

   $\frac{1}{x^{2} - a^{2}}$ $1/(x^2-a^2)$ convert to $\frac{A}{x + a} + \frac{B}{x - a}$ $A/(x+a) + B/(x-a)$

   $\frac{p x + q}{(x + a) (x + b)}$ $(px+q)/((x+a)(x+b))$ $= \frac{A}{x + a}$ $=A/(x+a)$ $+ \frac{B}{x + b}$ $+ B/(x+b)$

   $\frac{p x + q}{{(x + a)}^{2}}$ $(px+q)/((x+a)^2)$ $= \frac{A}{x + a}$ $=A/(x+a)$ $+ \frac{B}{{(x + a)}^{2}}$ $+ B/((x+a)^2)$

   $\frac{p x^{2} + q x + r}{(x + a) (x + b) (x + c)}$ $(px^2+qx+r)/((x+a)(x+b)(x+c))$ $= \frac{A}{x + a}$ $= A/(x+a)$ $+ \frac{B}{x + b}$ $+ B/(x+b)$ $+ \frac{C}{x + c}$ $+ C/(x+c)$

   $\frac{p x^{2} + q x + r}{{(x + a)}^{2} (x + b)}$ $(px^2+qx+r)/((x+a)^2(x+b))$ $= \frac{A}{x + a}$ $=A/(x+a)$ $+ \frac{B}{{(x + a)}^{2}}$ $+ B/((x+a)^2)$ $+ \frac{C}{x + b}$ $+ C/(x+b)$

   $\frac{p x^{2} + q x + r}{(x + a) (x^{2} + b x + c)}$ $(px^2+qx+r)/((x+a)(x^2+bx+c))$ $= \frac{A}{x + a}$ $=A/(x+a)$ $+ \frac{B x + C}{x^{2} + b x + c}$ $+ (Bx+C)/(x^2+bx+c)$

» Integration by Partial Fraction, when denominator cannot be factorized

   $\frac{1}{\sqrt{a x^{2} + b x + c}}$ $1/sqrt(ax^2+bx+c)$ convert denominator to $y^{2} \pm k^{2}$ $y^2+-k^2$ form

   $\frac{1}{a x^{2} + b x + c}$ $1/(ax^2+bx+c)$ convert denominator to $y^{2} \pm k^{2}$ $y^2+-k^2$ form

   $\frac{p x + q}{a x^{2} + b x + c}$ $(px+q)/(ax^2+bx+c)$ convert to two terms, first with numerator as derivative of denominator + second with a constant by denominator
$\frac{(a x^{2} + b x + c)'}{a x^{2} + b x + c}$ $((ax^2+bx+c)′)/(ax^2+bx+c)$ $+ \frac{A}{a x^{2} + b x + c}$ $+ A/(ax^2+bx+c)$

   $\frac{p x + q}{\sqrt{a x^{2} + b x + c}}$ $(px+q)/sqrt(ax^2+bx+c)$ convert to two terms, first with numerator as derivative of the quadratic equation + second with a constant by denominator
$\frac{(a x^{2} + b x + c)'}{\sqrt{a x^{2} + b x + c}}$ $((ax^2+bx+c)′)/sqrt(ax^2+bx+c)$ $+ \frac{A}{\sqrt{a x^{2} + b x + c}}$ $+ A/sqrt(ax^2+bx+c)$

maths > integral-calculus > integration-combination-of-methods

Integration by Combination of Methods

In this page, integration with combination of methods learned is introduced.