Decimals : Conversion of Repeating Decimals to Fractions

maths > decimals

Conversion of Repeating Decimals to Fractions

what you'll learn...

overview

This page covers

• Some fractions result in decimals with repeating digits.

• Representation of Repeating Decimals.

• Conversion of Repeating Decimals to equivalent Fractions : A decimal has two parts, a non-repeating part at the beginning and repetitive part. With some simple arithmetics, equivalent fraction is derived.

eg: $0 . \bar{3}$ $0.bar(3)$ is represented as $10 x - x = 3 . \bar{3} - 0 . \bar{3}$ $10x-x = 3.bar(3) - 0.bar(3)$ . The value of $x$ $x$ is derived as fraction $\frac{1}{3}$ $1/3$ .

never ending...

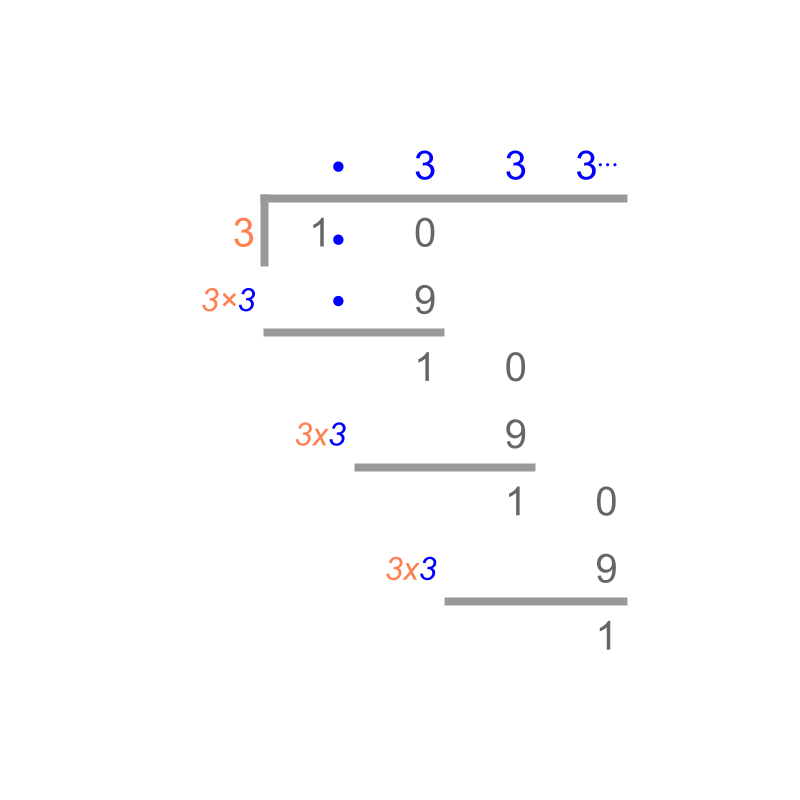
Convert $\frac{1}{3}$ $1/3$ into a decimal. The answer is " $0.333 \dots$ $0.333cdots$ ". It is noted that the decimal number does not end and number $3$ $3$ repeats. The long-division method to convert the fraction into a decimal is illustrated in the figure.

Convert $\frac{23}{9}$ $23/9$ into a decimal.
The answer is " $2.555 \dots$ $2.555cdots$ ".
Note: $\dots$ $cdots$ is used to represent that the digits are repeating.

Convert $\frac{371}{990}$ $371/990$ into a decimal.
The answer is " $0.37474 \dots$ $0.37474cdots$ ". It is noted that the decimal number does not end and number $7$ $7$ and $4$ $4$ repeats.

representing the repetition

$\frac{371}{990} = 0.37474 \dots$ $371/990 = 0.37474cdots$

In the above representation with $\dots$ $cdots$ , it is not clear which part of the digits are repeating

• is $4$ $4$ repeated? like $0.37474444444 \dots$ $0.37474444444cdots$

• is $74$ $74$ repeated? like $0.37474747474 \dots$ $0.37474747474cdots$

• is $37474$ $37474$ repeated? like $0.3747437474 \dots$ $0.3747437474cdots$

To avoid the confusion the following representation is adapted. The number is given as
$0.3 \bar{74}$ $0.3bar(74)$

The line over $74$ $74$ represents that $74$ $74$ is repeated.

Convert $\frac{1}{3}$ $1/3$ into a fraction.
The answer is $0 . \bar{3}$ $0.bar(3)$

Representation of Repeating Decimals : The repetitive pattern in decimal digits is represented with an over-line.

convert back to fraction

How do we convert $0 . \bar{4}$ $0.bar(4)$ into a fraction?

To convert $0 . \bar{4}$ $0.bar(4)$ into a fraction, the following steps are used

$x = 0 . \bar{4}$ $x=0.bar(4)$
$10 x = 4 . \bar{4}$ $10x = 4.bar(4)$

Subtracting the two equations
$9 x = 4$ $9x = 4$
$x = \frac{4}{9}$ $x=4/9$

examples

Converting $2 . \bar{4}$ $2.bar(4)$ into a fraction.
$x = 2 . \bar{4}$ $x=2.bar(4)$
$10 x = 24 . \bar{4}$ $10x = 24.bar(4)$

subtracting the two above
$9 x = 22$ $9x = 22$
$x = \frac{22}{9}$ $x=22/9$
$x = 2 \frac{4}{9}$ $x=2 4/9$

To convert $2.23 \bar{43}$ $2.23bar(43)$ into a fraction:

$x = 2.23 \bar{43}$ $x=2.23bar(43)$
$100 x = 223.43 \bar{43}$ $100x = 223.43bar(43)$
Subtracting the above,
$99 x = 221.20$ $99x = 221.20$
$990 x = 2212$ $990x = 2212$
$x = \frac{2212}{990}$ $x = 2212/990$ .

Another method is as follows.
$100 x = 223 + 0 . \bar{43}$ $100x = 223 + 0.bar(43)$
$10000 x = 223 \times 100 + 43 . \bar{43}$ $10000x =223xx100 + 43.bar(43)$
Subtracting the two equations
$9900 x = 223 \times (100 - 1) + 43$ $9900x = 223xx (100-1) + 43$
$x = \frac{223}{100} + \frac{43}{9900}$ $x=223/100 + 43/9900$

summary

Representation of Repeating Decimals : The repetitive pattern in decimal digits is represented with an over-line.

Conversion of Repeating Decimals to equivalent Fractions : A decimal has two parts, a non-repeating part at the beginning and repetitive part. With some simple arithmetics, equivalent fraction is derived.

eg: $0 . \bar{3}$ $0.bar(3)$ is represented as $10 x - x = 3 . \bar{3} - 0 . \bar{3}$ $10x-x = 3.bar(3) - 0.bar(3)$ . The value of $x$ $x$ is derived as fraction $\frac{1}{3}$ $1/3$ .

Outline
The outline of material to learn "decimals" is as follows.

Note: goto detailed outline of Decimals

• Decimals - Introduction

→ Decimals as Standard form of Fractions

→ Expanded form of Decimals

• Decimals - Conversion

→ Conversion between decimals and fractions

→ Repeating decimals

→ Irrational Numbers

• Decimals - Arithmetics

→ Comparing decimals

→ Addition & Subtraction

→ Multiplication

→ Division

• Decimals - Expressions

→ Expression Simplification

→ PEMA / BOMA