Decimals : Decimal Division

maths > decimals

Decimal Division

what you'll learn...

overview

Decimals are fractions with standard place values. In this page, the following for decimals are explained.

• Division in first principles -- splitting a quantity into a number of parts and measuring one part

• simplified procedure : Sign property of division and long division method for Decimals.

recap

"Division" can be described as

dividend split into divisor number of parts.

$11 \div 4 =$ $11 -: 4 =$ $2$ $2$ quotient and $3$ $3$ remainder. Considering the figure with $11$ $11$ cars and $4$ $4$ dotted-boxes. $11$ $11$ is split into $4$ $4$ equal parts with each assigned $2$ $2$ cars. There are $3$ $3$ cars remaining. The remaining cars are not enough to assign equally to each dotted-box.

One part is counted as $2$ $2$ and remaining amount is $3$ $3$ .

division is inverse of multiplication. And division of fractions are explained with that.

$\frac{3}{4} \div \frac{2}{3}$ $3/4 -: 2/3$
$= \frac{3}{4} \times \frac{3}{2}$ $quad = 3/4 xx 3/2$ (multiply by inverse)
$= \frac{9}{8}$ $quad = 9/8$ .

splitting

Decimals are fractions with standardized place-values.

Consider $0.6 \div 3 = 0.2$ $0.6 -: 3 = 0.2$ .

By first principles, the dividend $0.6 = \frac{6}{10}$ $0.6 = 6/10$ is split into divisor $3$ $3$ number of equal parts : $\frac{2}{10}; \frac{2}{10}; \frac{2}{10}$ $2/10 ; 2/10 ; 2/10$ . And one part is $\frac{2}{10}$ $2/10$ , or equals $0.2$ $0.2$ in decimals.

Consider $0.6 \div 0.3 = 2$ $0.6 -: 0.3 = 2$ ".

By first principles, the dividend $0.6 = \frac{6}{10}$ $0.6 = 6/10$ is split into divisor $0.3 = \frac{3}{10}$ $0.3 = 3/10$ number of equal parts :

This is done in two steps:
first step: $\frac{6}{10}$ $6/10$ is split into $\frac{1}{10}$ $1/10$ (denominator of $\frac{3}{10}$ $3/10$ ) times is $\frac{60}{10}$ $60/10$ .
Second step: the result from the first step $\frac{60}{10}$ $60/10$ is split into $3$ $3$ (numerator of $\frac{3}{10}$ $3/10$ ) times is $\frac{20}{10}$ $20/10$

The result is $\frac{20}{10}$ $20/10$ , or equals $2$ $2$ in decimals.

aligned or opposed to direction

Decimals are directed numbers, that is decimals are either positive or negative.

$0.2$ $0.2$ aligned in direction is " $+ 0.2$ $+0.2$ ".

$0.2$ $0.2$ opposed in direction is " $- 0.2$ $-0.2$ ".

Directed numbers, positive and negative, are explained as "aligned in direction" and "opposed in direction" respectively.

Consider $0.6 \div (- 0.3) = - 2$ $0.6 -: (-0.3) = -2$

By first principles, the dividend $0.6 = \frac{6}{10}$ $0.6 = 6/10$ aligned in direction is split into the divisor $0.3 = \frac{3}{10}$ $0.3=3/10$ opposed in direction number of equal parts :

This is done in two steps,
first step: $\frac{6}{10}$ $6/10$ aligned in direction is split into $\frac{1}{10}$ $1/10$ aligned in direction (denominator of $\frac{3}{10}$ $3/10$ ) times is $\frac{60}{10}$ $60/10$ aligned in direction.
Second step: the result from the first step $\frac{60}{10}$ $60/10$ aligned in direction is split into $3$ $3$ (numerator of $\frac{3}{10}$ $3/10$ )opposed in direction times is $\frac{20}{10}$ $20/10$ opposed in direction.

The result is $\frac{20}{10}$ $20/10$ opposed in direction, or equals $- 2$ $-2$ in decimal number form.

decimal division

Decimal Division by first principle : Decimal division is splitting the dividend, into divisor number of parts with sign of the numbers (direction) handled appropriately.

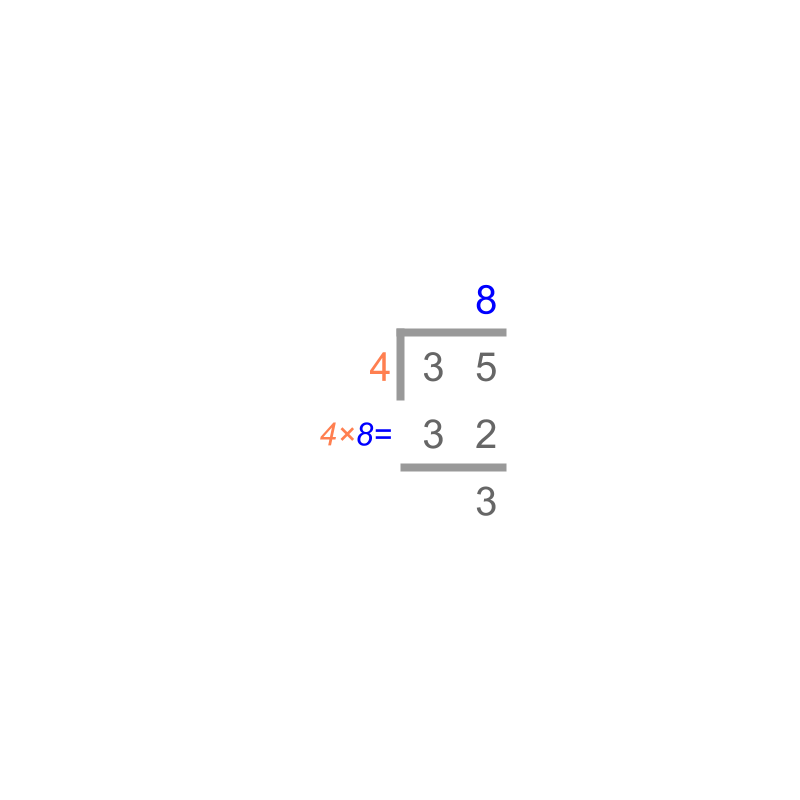
In whole numbers, we have studied Division by Place-value as illustrated in the figure. This procedure is used in decimals in a later step.

In Integers, we have studied Sign-property of Division.
• +ve $\div$ $-:$ +ve = +ve
• +ve $\div$ $-:$ -ve = -ve
• -ve $\div$ $-:$ +ve = -ve
• -ve $\div$ $-:$ -ve = +ve
This is applicable to decimals.

In Fractions, we have studied that division is inverse of multiplication.
For example, to divide $\frac{4}{5} \div \frac{3}{2}$ $4/5 -: 3/2$ , it is modified to $\frac{4}{5} \times \frac{2}{3}$ $4/5 xx 2/3$

Decimals are divided keeping in mind the place-value representation, which is a form of fractions.
For example, to divide $1.8 \div .06$ $1.8 -: .06$ , it is equivalently thought as $\frac{18}{10} \div \frac{6}{100}$ $18/10 -: 6/100$ and modified to multiplication $\frac{18}{10} \times \frac{100}{6}$ $18/10 xx 100/6$ .

simplify

Consider division of $0.002 \div 0.05$ $0.002 -: 0.05$

This is equivalently $\frac{2}{1000} \div \frac{5}{100}$ $2/1000 -: 5/100$
$= \frac{2}{1000} \times \frac{100}{5}$ $=2/1000 xx 100/5$
$= \frac{2 \times 100}{1000 \times 5}$ $=(2xx100)/(1000xx 5)$
$= \frac{2}{50}$ $=2/50$
$= 0.04$ $=0.04$

Understanding the above, a simplified procedure to divide the decimals is devised.

The decimal point of dividend and divisor are removed and the numbers are divided as integers.
eg: $0.002$ $0.002$ is modified to the integer form $2$ $2$ .
$0.05$ $0.05$ is modified to the integer form $5$ $5$ .

The number of decimal-places in the dividend and divisor are counted.
eg: $0.002$ $0.002$ has $3$ $3$ decimal-places.
$0.05$ $0.05$ has $2$ $2$ decimal-places.

Now the integer forms are divided.
eg: $2 \div 5 = 0.4$ $2-:5 = 0.4$

The number of decimal places of divisor is subtracted from dividend number of decimal places.
eg: $3 - 2 = 1$ $3-2=1$ .

The quotient form is modified to have the number of decimal points give by the difference above.
eg: $0.4$ $0.4$ is modified to $0.04$ $0.04$ , that is decimal point moved to the left by $1$ $1$ .

example

Consider $1.55 \div .005 = 310$ $1.55 -: .005 = 310$ ".

$155 \div 5 = 31 .$ $155-: 5 = 31.$

Difference in decimal places is $2 - 3 = - 1$ $2-3=-1$

So the product decimal place moves $1$ $1$ place to the right.
$1.55 \div 0.005 = 310$ $1.55 -: 0.005 = 310$

summary

Decimal Division -- Simplified Procedure :
The signs (+ve / -ve) are handled as in Sign-property of Integer Division
• +ve $\div$ $-:$ +ve = +ve
• +ve $\div$ $-:$ -ve = -ve
• -ve $\div$ $-:$ +ve = -ve
• -ve $\div$ $-:$ -ve = +ve

The decimal places are removed and the division is carried out as per Whole number Division by Place Value.

• The decimal-point is modified in the result
• The decimal-point is moved to the left the number of times equal to the difference number of decimals in dividend minus number of decimals in divisor. A positive difference moves the decimal point to the left, and a negative difference moves the decimal point to the right.

Outline

The outline of material to learn "decimals" is as follows.

Note: goto detailed outline of Decimals

• Decimals - Introduction

→ Decimals as Standard form of Fractions

→ Expanded form of Decimals

• Decimals - Conversion

→ Conversion between decimals and fractions

→ Repeating decimals

→ Irrational Numbers

• Decimals - Arithmetics

→ Comparing decimals

→ Addition & Subtraction

→ Multiplication

→ Division

• Decimals - Expressions

→ Expression Simplification

→ PEMA / BOMA