Algebra : Foundation with Numerical Arithmetics : Laws and Properties of Arithmetics : Multiplication

maths > algebra

Laws and Properties of Arithmetics : Multiplication

what you'll learn...

Overview

CADI Properties of Multiplication

» Closure Property
    → if $x, y \in ℝ$ $x,y in RR$ , then $x \times y \in ℝ$ $x xx y in RR$

» Commutative Property
    → $x \times y = y \times x$ $x xx y = y xx x$

» Associative Property
    → $(x \times y) \times z = x \times (y \times z)$ $(x xx y) xx z = x xx (y xx z)$

» Distributive Property Over Addition
    → $(x + y) \times z = x \times z + y \times z$ $(x + y)xx z = x xx z + y xx z$

» Multiplicative Identity Property
    → $1 \in ℝ$ $1 in RR$ , such that $x \times 1 = x$ $x xx 1 = x$

» Additive Inverse Property
    → $\frac{1}{x} \in ℝ$ $1/x in RR$ for any $x \in ℝ$ $x in RR$ (except for $0$ $0$ ) such that $x \times (\frac{1}{x}) = 1$ $x xx (1/x) = 1$

» Division is to be handled as multiplicative inverse for the properties
this is important as algebra extensively involves these properties
    → Commutative property involving division : $x \div y$ $x -: y$ is given as $x \times \frac{1}{y} = \frac{1}{y} \times x$ $x xx 1/y = 1/y xx x$
    → Associative property involving division : $(x \div y) \div z$ $(x-:y)-:z$ is given as $(x \times \frac{1}{y}) \times \frac{1}{z} = x \times (\frac{1}{y} \times \frac{1}{z})$ $(x xx 1/y) xx 1/z = x xx (1/y xx 1/z)$
    → Distributive property involving division : $p \div q \times (x + y)$ $p-:q xx (x+y)$ is given as $p \times \frac{1}{q} \times (x + y) = p \times (\frac{x}{q} + \frac{y}{q})$ $p xx 1/q xx (x+y) = p xx (x/q + y/q)$

In this lesson, the laws and properties of multiplication is revised. It is very important to go through this once to understand algebra.

closure means within

For any real numbers $p, q \in ℝ$ $p,q in RR$ , $p \times q$ $p xx q$ is a real number.

Closure Property of Multiplication: Given $p, q \in ℝ$ $p,q in RR$ . $p \times q \in ℝ$ $p xx q in RR$ .

Closure Property applied to Division: Given $p, q \in ℝ$ $p,q in RR$ . $p \div q \in ℝ$ $p-:q in RR$ .

Proof:
Given $p, q \in ℝ$ $p,q in RR$
$\frac{1}{q} \in ℝ$ $1/q in RR$ as per Multiplicative Inverse Property
$p \times (\frac{1}{q}) \in ℝ$ $pxx(1/q) in RR$ as per Closure property of Multiplication
$\Rightarrow p \div q \in ℝ$ $=> p-:q in RR$

Using Closure Property: Given $p, q, r, s \in ℝ$ $p,q,r,s in RR$ , $p \times q \div r \times s$ $pxxq-:rxxs$ , the subexpression $p \times q \div r$ $pxxq-:r$ is a number and can be considered as a single number for any other property.

For example as per commutative property $p \times q \div r \times s = s \times p \times q \div r$ $pxxq-:rxxs = sxxpxxq-:r$ , in which $p \times q \div r$ $pxxq-:r$ is considered to be a single real number.

forward and backward

Given $p, q \in ℝ$ $p,q in RR$ . $p \times q = q \times p$ $pxxq = qxxp$

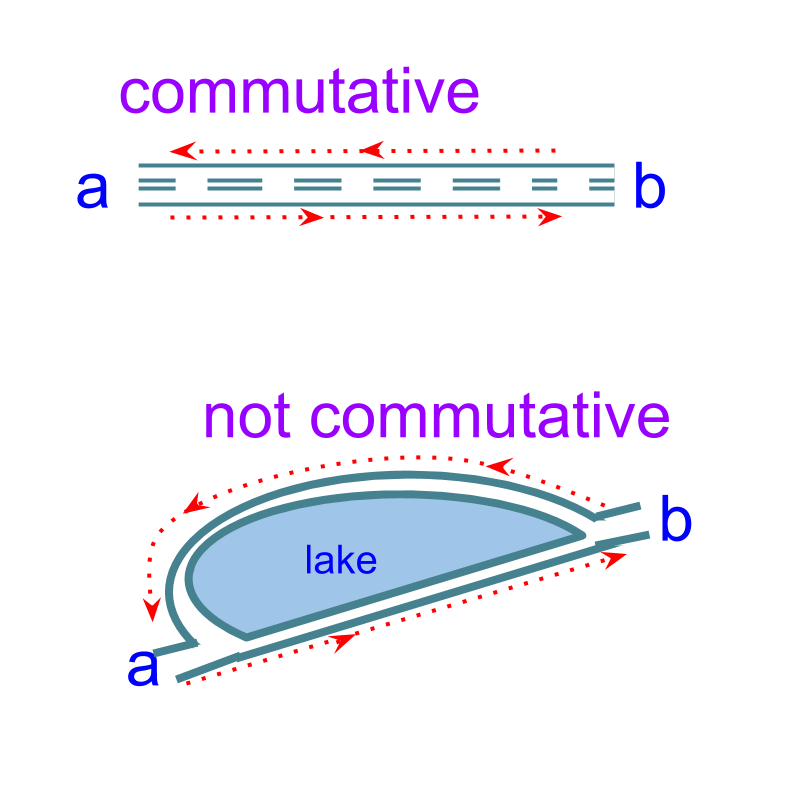
Commutative Property of Multiplication: Given $p, q \in ℝ$ $p,q in RR$ , $p \times q = q \times r$ $pxxq=qxxr$ .

Commutative Property applied to Division: $p \div q = \frac{1}{q} \times p$ $p-:q=1/qxxp$ .
Note: Division has to be handled as inverse of multiplication, $p \div q = p \times (\frac{1}{q})$ $p-:q = pxx(1/q)$ and then commutative property can be used.

Using Commutative Property: Given $p, q, r \in ℝ$ $p,q,r in RR$ , the expression $p \times q \times p \div q \times r \div p \times r^{2}$ $pxxqxxp-:qxxr-:pxxr^2$ is simplified to $p r^{3}$ $pr^3$ . students may work this out to understand.

with this or that

Given $p, q, r \in ℝ$ $p,q,r in RR$ . $(p \times q) \times r = p \times (q \times r)$ $(pxxq)xxr = pxx(qxxr)$

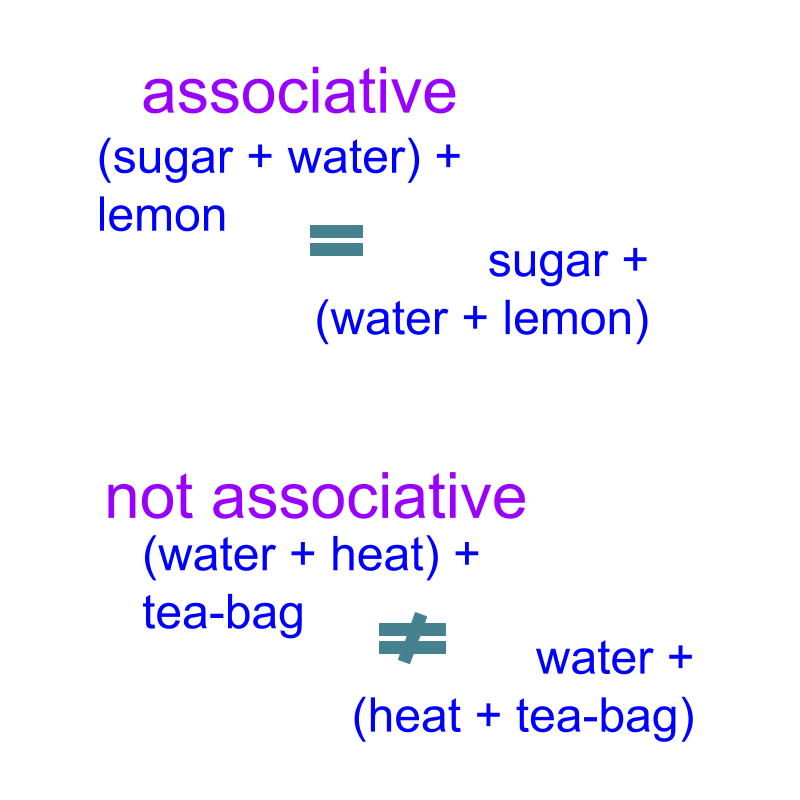
Associative Property of Multiplication: Given $p, q, r \in ℝ$ $p,q,r in RR$ . $(p \times q) \times r = p \times (q \times r)$ $(pxxq)xxr = pxx(qxxr)$ .

Associative Property applied to Division:

$(p \div q) \div r = p \times (\frac{1}{q} \times \frac{1}{r})$ $(p-:q)-:r = pxx(1/q xx 1/r)$ .
Note: Division has to be handled as inverse of multiplication, $(p \div q) \div r = (p \times \frac{1}{q}) \times \frac{1}{r}$ $(p-:q)-:r = (pxx 1/q)xx 1/r$ and then associative property can be used.

spread across

Given $p, q, r \in ℝ$ $p,q,r in RR$ . Which of the following equals $(p + q) \times r = p \times r + q \times r$ $(p+q)xxr = pxxr + q xx r$

The word 'distribute' means 'to share; to spread'

Distributive Property of Multiplication: Given $p, q, r \in ℝ$ $p,q,r in RR$ . $(p + q) \times r = p \times r + q \times r$ $(p+q)xxr = pxxr + q xx r$ .

Distributive Property applied to Division: There are 3 possible scenarios. In any such scenario, convert the division to inverse of multiplication, and distributive property applies.

(1) $(p + q) \div r = \frac{p}{r} + \frac{q}{r}$ $(p+q)-:r = p/r + q/r$ .

(2) $r \div (p + q) = \frac{r}{p + q}$ $r-:(p+q) = r/(p+q)$ . The multiplication does not distribute in this case.

(3) $t \div r \times (p + q) = t \times (\frac{p}{r} + \frac{q}{r})$ $t-:r xx (p+q) = t xx (p/r + q/r)$ .

In algebra, division is always given as $\frac{p}{q}$ $p/q$ and not $p \div q$ $p-:q$ . Thus commutative property, associative property, and distributive property can be used without any unintended errors.

one

Given $p \in ℝ$ $p in RR$ . What is $p \times 1 = p$ $pxx1=p$

Multiplicative Identity Property: For any $p \in ℝ$ $p in RR$ , there exists $1 \in ℝ$ $1 in RR$ such that $p \times 1 = p$ $pxx1=p$ .

Multiplicative Identity applied to Division: $p \div 1 = p$ $p-:1 = p$

Note: $\frac{1}{1} = 1$ $1/1=1$ and so $p \div 1 = p \times (\frac{1}{1}) = p \times 1 = p$ $p-:1=pxx(1/1)=pxx1 = p$ .

inverse

Given $p \in ℝ$ $p in RR$ . What is $p \times \frac{1}{p}$ $pxx 1/p$ ?

Multiplicative Inverse Property: For any $p \in ℝ$ $p in RR$ and $p \neq 0$ $p!=0$ , there exists $\frac{1}{p} \in ℝ$ $1/p in RR$ such that $p \times \frac{1}{p} = 1$ $pxx 1/p=1$ .

summary

The properties together are named as CADI properties of multiplication. The abbreviation CADI is a simplified form of the first letters of Closure, Commutative, Associative, Distributive, Inverse, and Identity properties.

Note: Distributive property is shared with addition.

The CADI properties of Addition and CADI properties of Multiplication are together referred as "CADI properties".

LPA: CADI properties of Multiplication
• Closure Property
      if $x, y \in ℝ$ $x,y in RR$ , then $x \times y \in ℝ$ $x xx y in RR$

• Commutative Property
      $x \times y = y \times x$ $x xx y = y xx x$

• Associative Property
      $(x \times y) \times z = x \times (y \times z)$ $(x xx y) xx z = x xx (y xx z)$

• Distributive Property Over Addition
      $(x + y) \times z = x \times z + y \times z$ $(x + y)xx z = x xx z + y xx z$

• Multiplicative Identity Property
      $1 \in ℝ$ $1 in RR$ , such that $x \times 1 = x$ $x xx 1 = x$

• Multiplicative Inverse Property
      $\frac{1}{x} \in ℝ$ $1/x in RR$ for any $x \in ℝ$ $x in RR$ (except for $0$ $0$ ) such that $x \times (\frac{1}{x}) = 1$ $x xx (1/x) = 1$

• Division is to be handled as inverse of multiplication for the properties

This is important as algebra extensively involves these properties.

      Commutative property involving division : $x \div y$ $x -: y$ is given as $x \times \frac{1}{y} = \frac{1}{y} \times x$ $x xx 1/y = 1/y xx x$

      Associative property involving division : $(x \div y) \div z$ $(x-:y)-:z$ is given as $(x \times \frac{1}{y}) \times \frac{1}{z} = x \times (\frac{1}{y} \times \frac{1}{z})$ $(x xx 1/y) xx 1/z = x xx (1/y xx 1/z)$

      Distributive property involving division : $p \div q \times (x + y)$ $p-:q xx (x+y)$ is given as $p \times \frac{1}{q} \times (x + y) = p \times (\frac{x}{q} + \frac{y}{q})$ $p xx 1/q xx (x+y) = p xx (x/q + y/q)$

Outline

The outline of material to learn "Algebra Foundation" is as follows.

Note: click here for detailed outline of Foundation of Algebra

→ Numerical Arithmetics

→ Arithmetic Operations and Precedence

→ Properties of Comparison

→ Properties of Addition

→ Properties of Multiplication

→ Properties of Exponents

→ Algebraic Expressions

→ Algebraic Equations

→ Algebraic Identities

→ Algebraic Inequations

→ Brief about Algebra